Lista över enhetliga polyedrar

Inom geometri är en enhetlig polyeder en polyeder som har regelbundna polygoner som vänder mot och är vertextransitiv ( transitiv på sina hörn , isogonal, dvs. det finns en isometri som kartlägger vilken vertex som helst på någon annan). Det följer att alla hörn är kongruenta och polyedern har en hög grad av reflektions- och rotationssymmetri .

Enhetliga polyedrar kan delas mellan konvexa former med konvexa regelbundna polygonytor och stjärnformer. Stjärnformer har antingen vanliga stjärnpolygonytor eller vertexfigurer eller båda .

Den här listan innehåller dessa:

• alla 75 icke-prismatiska enhetliga polyedrar ;
• några få representanter för de oändliga uppsättningarna av prismor och antiprismor ;
• en degenererad polyeder, Skillings figur med överlappande kanter.

Det bevisades i Sopov (1970) att det bara finns 75 enhetliga polyedrar förutom de oändliga familjerna av prismor och antiprismor . John Skilling upptäckte ett förbisett degenererat exempel, genom att slappna av tillståndet att endast två ansikten kan mötas vid en kant. Detta är en degenererad enhetlig polyeder snarare än en enhetlig polyeder, eftersom vissa par av kanter sammanfaller.

Ej inkluderat är:

• De enhetliga polyederföreningarna .
• 40 potentiella enhetliga polyedrar med degenererade vertexfigurer som har överlappande kanter (räknas inte av Coxeter );
• De enhetliga plattorna (oändliga polyedrar)
  • 11 Euklidiska konvexa likformiga plattsättningar ;
  • 28 Euklidiska icke-konvexa eller apeirogonala enhetliga plattor ;
  • Oändligt antal enhetliga plattsättningar i hyperboliskt plan .
• Alla polygoner eller 4-polytoper

Indexering

Fyra numreringsscheman för de enhetliga polyedrarna är i vanligt bruk, kännetecknade av bokstäver:

• [ C ] Coxeter et al., 1954, visade de konvexa formerna som figurerna 15 till 32; tre prismatiska former, figurerna 33–35; och de icke-konvexa formerna, figurerna 36–92.
• [ W ] Wenninger, 1974, har 119 siffror: 1–5 för de platonska soliderna, 6–18 för de arkimedeiska soliderna, 19–66 för stellerade former inklusive de 4 reguljära icke-konvexa polyedrarna, och slutade med 67–119 för den icke-konvexa uniformen polyedrar.
• [ K ] Kaleido, 1993: De 80 figurerna grupperades efter symmetri: 1–5 som representanter för de oändliga familjerna av prismatiska former med dihedrisk symmetri , 6–9 med tetraedrisk symmetri , 10–26 med oktaedrisk symmetri , 27–80 med symmetri .
• [ U ] Mathematica, 1993, följer Kaleido-serien med de 5 prismatiska formerna flyttade till att hålla, så att de ickeprismatiska formerna blir 1–75.

Namn på polyedrar efter antal sidor

Det finns generiska geometriska namn för de vanligaste polyedrarna . De 5 platoniska fasta ämnena kallas en tetraeder , hexahedron , oktaeder , dodekaeder och icosahedron med 4, 6, 8, 12 respektive 20 sidor. Den vanliga hexaedern är en kub .

Tabell av polyedrar

De konvexa formerna är listade i ordning efter grad av vertexkonfigurationer från 3 ytor/vertex och uppåt, och i ökande sidor per sida. Denna ordning gör att topologiska likheter kan visas.

Det finns oändligt många prismor och antiprismor, en för varje vanlig polygon; de upp till de 12-gonala fallen listas.

Konvexa enhetliga polyedrar

namn	Bild	Vertex typ	Wythoff symbol	Sym.	C#	W#	U#	K#	Vert.	Kanter	Ansikten	Ansikten efter typ
Tetraeder		3.3.3	3 | 2 3	T d	C15	W001	U01	K06	4	6	4	4{3}
Trekantsprisma		3.4.4	2 3 | 2	D 3h	C33a	—	U76a	K01a	6	9	5	2{3} +3{4}
Trunkerad tetraeder		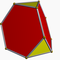3.6.6	2 3 | 3	T d	C16	W006	U02	K07	12	18	8	4{3} +4{6}
Stympad kub		3.8.8	2 3 | 4	O h	C21	W008	U09	K14	24	36	14	8{3} +6{8}
Stympad dodekaeder		3.10.10	2 3 | 5	jag h	C29	W010	U26	K31	60	90	32	20{3} +12{10}
Kub		4.4.4	3 | 2 4	O h	C18	W003	U06	K11	8	12	6	6{4}
Pentagonal prisma		4.4.5	2 5 | 2	D 5h	C33b	—	U76b	K01b	10	15	7	5{4} +2{5}
Sexkantigt prisma		4.4.6	2 6 | 2	D 6h	C33c	—	U76c	K01c	12	18	8	6{4} +2{6}
Heptagonalt prisma		4.4.7	2 7 | 2	D 7h	C33d	—	U76d	K01d	14	21	9	7{4} +2{7}
Åttakantigt prisma		4.4.8	2 8 | 2	D 8h	C33e	—	U76e	K01e	16	24	10	8{4} +2{8}
Enneagonal prisma		4.4.9	2 9 | 2	D 9h	C33f	—	U76f	K01f	18	27	11	9{4} +2{9}
Dekagonalt prisma		4.4.10	2 10 | 2	D 10h	C33g	—	U76g	K01g	20	30	12	10{4} +2{10}
Hendekagonalt prisma		4.4.11	2 11 | 2	D 11h	C33h	—	U76h	K01h	22	33	13	11{4} +2{11}
Dodekagonalt prisma		4.4.12	2 12 | 2	D 12h	C33i	—	U76i	K01i	24	36	14	12{4} +2{12}
Stympad oktaeder		4.6.6	2 4 | 3	O h	C20	W007	U08	K13	24	36	14	6{4} +8{6}
Stympad cuboctahedron		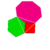4.6.8	2 3 4 |	O h	C23	W015	U11	K16	48	72	26	12{4} +8{6} +6{8}
Stympad icosidodecahedron		4.6.10	2 3 5 |	jag h	C31	W016	U28	K33	120	180	62	30{4} +20{6} +12{10}
Dodekaeder		5.5.5	3 | 2 5	jag h	C26	W005	U23	K28	20	30	12	12{5}
Stympad icosahedron		5.6.6	2 5 | 3	jag h	C27	W009	U25	K30	60	90	32	12{5} +20{6}
Oktaeder		3.3.3.3	4 | 2 3	O h	C17	W002	U05	K10	6	12	8	8{3}
Fyrkantig antiprisma		3.3.3.4	| 2 2 4	D 4d	C34a	—	U77a	K02a	8	16	10	8{3} +2{4}
Pentagonal antiprisma		3.3.3.5	| 2 2 5	D 5d	C34b	—	U77b	K02b	10	20	12	10{3} +2{5}
Hexagonal antiprisma		3.3.3.6	| 2 2 6	D 6d	C34c	—	U77c	K02c	12	24	14	12{3} +2{6}
Heptagonal antiprisma		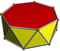3.3.3.7	| 2 2 7	D 7d	C34d	—	U77d	K02d	14	28	16	14{3} +2{7}
Octagonal antiprisma		3.3.3.8	| 2 2 8	D 8d	C34e	—	U77e	K02e	16	32	18	16{3} +2{8}
Enneagonal antiprisma		3.3.3.9	| 2 2 9	D 9d	C34f	—	U77f	K02f	18	36	20	18{3} +2{9}
Dekagonal antiprisma		3.3.3.10	| 2 2 10	D 10d	C34g	—	U77g	K02g	20	40	22	20{3} +2{10}
Hendekagonal antiprisma		3.3.3.11	| 2 2 11	D 11d	C34h	—	U77h	K02h	22	44	24	22{3} +2{11}
Dodekagonal antiprisma		3.3.3.12	| 2 2 12	D 12d	C34i	—	U77i	K02i	24	48	26	24{3} +2{12}
Cuboctahedron		3.4.3.4	2 | 3 4	O h	C19	W011	U07	K12	12	24	14	8{3} +6{4}
Rhombicuboctahedron		3.4.4.4	3 4 | 2	O h	C22	W013	U10	K15	24	48	26	8{3} +(6+12){4}
Rhombicosidodecahedron		3.4.5.4	3 5 | 2	jag h	C30	W014	U27	K32	60	120	62	20{3} +30{4} +12{5}
Icosidodecahedron		3.5.3.5	2 | 3 5	jag h	C28	W012	U24	K29	30	60	32	20{3} +12{5}
Icosahedron		3.3.3.3.3	5 | 2 3	jag h	C25	W004	U22	K27	12	30	20	20{3}
Snub kub		3.3.3.3.4	| 2 3 4	O	C24	W017	U12	K17	24	60	38	(8+24){3} +6{4}
Snub dodekaeder		3.3.3.3.5	| 2 3 5	jag	C32	W018	U29	K34	60	150	92	(20+60){3} +12{5}

Uniform stjärnpolyeder

Formerna som endast innehåller konvexa ytor listas först, följt av formulären med stjärnytor. Återigen finns det oändligt många prismor och antiprismor; de är listade här upp till de 8-sidiga.

De enhetliga polyedrarna | 5 / 2 3 3, | 5 / 2 3 / 2 3 / 2 , | 5 / 3 5 / 2 3, | 3/2 _ _ _ 5/3 3 _ 5/2 och | _ ( 3 / 2 ) 5 / 3 (3) 5 / 2 har några ytor som förekommer som par i samma plan. (Coxeter et al. 1954, s. 423, 425, 426; Skilling 1975, s. 123)

namn	Bild	Wyth sym	Vert. fikon	Sym.	C#	W#	U#	K#	Vert.	Kanter	Ansikten	Chi	Orienterbar ?	Dens.	Ansikten efter typ
Octahemioctahedron		3 / 2 3 | 3	6. 3 / 2 .6.3	O h	C37	W068	U03	K08	12	24	12	0	Ja		8{3}+4{6}
Tetrahemihexahedron		3 / 2 3 | 2	4. 3 / 2 .4.3	T d	C36	W067	U04	K09	6	12	7	1	Nej		4{3}+3{4}
Kubohemioctahedron		4 / 3 4 | 3	6. 4 / 3 .6.4	O h	C51	W078	U15	K20	12	24	10	−2	Nej		6{4}+4{6}
Stor dodekaeder		5/2 _ _ | 2 5	(5.5.5.5.5)/2	jag h	C44	W021	U35	K40	12	30	12	−6	Ja	3	12{5}
Stor ikosaeder		5/2 _ _ | 2 3	(3.3.3.3.3)/2	jag h	C69	W041	U53	K58	12	30	20	2	Ja	7	20{3}
Stor ditrigonal icosidodecahedron		3/2 _ _ | 3 5	(5.3.5.3.5.3)/2	jag h	C61	W087	U47	K52	20	60	32	−8	Ja	6	20{3}+12{5}
Liten rhombihexahedron		2 4 ( 3 / 2 4 / 2 ) |	4.8. 4/3 _ _ . 8/7 _ _	O h	C60	W086	U18	K23	24	48	18	−6	Nej		12{4}+6{8}
Liten cubicuboctahedron		3 / 2 4 | 4	8. 3/2 .8.4 _ _	O h	C38	W069	U13	K18	24	48	20	−4	Ja	2	8{3}+6{4}+6{8}
Stor rhombicuboctahedron		3 / 2 4 | 2	4. 3 / 2 .4.4	O h	C59	W085	U17	K22	24	48	26	2	Ja	5	8{3}+(6+12){4}
Liten dodekahemi- dodekaeder		5 / 4 5 | 5	10. 5 / 4 .10.5	jag h	C65	W091	U51	K56	30	60	18	−12	Nej		12{5}+6{10}
Stor dodecahem- ikosaeder		5 / 4 5 | 3	6. 5 / 4 .6.5	jag h	C81	W102	U65	K70	30	60	22	−8	Nej		12{5}+10{6}
Liten icosihemidodekaeder		3 / 2 3 | 5	10. 3 / 2 .10.3	jag h	C63	W089	U49	K54	30	60	26	−4	Nej		20{3}+6{10}
Liten dodecikosaeder		3 5 ( 3 / 2 5 / 4 ) |	10.6. 10/9 _ _ . 6/5 _ _	jag h	C64	W090	U50	K55	60	120	32	−28	Nej		20{6}+12{10}
Liten rhombidodecahedron		2 5 ( 3 / 2 5 / 2 ) |	10.4. 10/9 _ _ . 4/3 _ _	jag h	C46	W074	U39	K44	60	120	42	−18	Nej		30{4}+12{10}
Liten dodecicosi- dodekaeder		3 / 2 5 | 5	10. 3 / 2 .10.5	jag h	C42	W072	U33	K38	60	120	44	−16	Ja	2	20{3}+12{5}+12{10}
Rhombicosahedron		2 3 ( 5 / 4 5 / 2 ) |	6.4. 6/5 _ _ . 4/3 _ _	jag h	C72	W096	U56	K61	60	120	50	−10	Nej		30{4}+20{6}
Stor icosicosi- dodekaeder		3 / 2 5 | 3	6. 3/2 _ _ .6.5	jag h	C62	W088	U48	K53	60	120	52	−8	Ja	6	20{3}+12{5}+20{6}
Pentagrammisk prisma		2 5 / 2 | 2	5/2 .4.4 _ _	D 5h	C33b	—	U78a	K03a	10	15	7	2	Ja	2	5{4} +2 { 5/2 }
Heptagrammiskt prisma (7/2)		2 7 / 2 | 2	7/2 .4.4 _ _	D 7h	C33d	—	U78b	K03b	14	21	9	2	Ja	2	7{4} +2 { 7/2 }
Heptagrammiskt prisma (7/3)		2 7 / 3 | 2	7/3 .4.4 _ _	D 7h	C33d	—	U78c	K03c	14	21	9	2	Ja	3	7{4} +2 { 7/3 }
Oktagrammisk prisma		2 8 / 3 | 2	8/3 .4.4 _ _	D 8h	C33e	—	U78d	K03d	16	24	10	2	Ja	3	8{4} +2 { 8/3 }
Pentagrammisk antiprism		| 2 2 5/2 _	5/2 .3.3.3 _ _	D 5h	C34b	—	U79a	K04a	10	20	12	2	Ja	2	10{3} +2 { 5/2 }
Pentagrammisk korsad antiprism		| 2 2 5 / 3	5/3 .3.3.3 _ _	D 5d	C35a	—	U80a	K05a	10	20	12	2	Ja	3	10{3} +2 { 5/2 }
Heptagrammisk antiprisma (7/2)		| 2 2 7/2 _	7/2 .3.3.3 _ _	D 7h	C34d	—	U79b	K04b	14	28	16	2	Ja	3	14{3} +2 { 7/2 }
Heptagrammisk antiprisma (7/3)		| 2 2 7 / 3	7/3 .3.3.3 _ _	D 7d	C34d	—	U79c	K04c	14	28	16	2	Ja	3	14{3} +2 { 7/3 }
Heptagrammisk korsad antiprism		| 2 2 7 / 4	7/4 _ _ .3.3.3	D 7h	C35b	—	U80b	K05b	14	28	16	2	Ja	4	14{3} +2 { 7/3 }
Oktagrammisk antiprisma		| 2 2 8 / 3	8/3 .3.3.3 _ _	D 8d	C34e	—	U79d	K04d	16	32	18	2	Ja	3	16{3} +2 { 8/3 }
Oktagrammisk korsad antiprism		| 2 2 8/5 _	8/5 _ _ .3.3.3	D 8d	C35c	—	U80c	K05c	16	32	18	2	Ja	5	16{3} +2 { 8/3 }
Liten stjärnformad dodekaeder		5 | 2 5/2 _ _	( 5/2 _ _ ) 5	jag h	C43	W020	U34	K39	12	30	12	−6	Ja	3	12 { 5/2 } _
Stor stjärnformad dodekaeder		3 | 2 5/2 _ _	( 5 / 2 ) 3	jag h	C68	W022	U52	K57	20	30	12	2	Ja	7	12 { 5/2 } _
Ditrigonal dodeca- dodecahedron		3 | 5/3 _ _ 5	( 5 / 3 .5) 3	jag h	C53	W080	U41	K46	20	60	24	−16	Ja	4	12{5} 5/2 } +12 {
Liten ditrigonal icosidodecahedron		3 | 5/2 3 _ _	( 5 / 2 .3) 3	jag h	C39	W070	U30	K35	20	60	32	−8	Ja	2	20{3} +12 { 5/2 }
Stellat stympad hexaeder		2 3 | 4/3 _ _	8/3 _ _ . 8/3 .3 _ _	O h	C66	W092	U19	K24	24	36	14	2	Ja	7	8{3} +6 { 8/3 }
Stor rhombihexahedron		2 4 / 3 ( 3 / 2 4 / 2 ) |	4. 8/3 _ _ . 4/3 _ _ . 8/5 _ _	O h	C82	W103	U21	K26	24	48	18	−6	Nej		12{4} +6 { 8/3 }
Stor cubicuboctahedron		3 4 | 4/3 _ _	_ 8/3 .3 . 8/3 .4 _ _	O h	C50	W077	U14	K19	24	48	20	−4	Ja	4	8/3 } 8 {3} +6 {4}+6{
Stor dodekahemidodekaeder		5 / 3 5 / 2 | 5/3 _ _	10/3 _ _ . 5/3 _ _ . 10/3 _ _ . 5/2 _ _	jag h	C86	W107	U70	K75	30	60	18	−12	Nej		12 { 10/3 { 5/2 } _ } +6
Liten dodekahemikosaeder		5 / 3 5 / 2 | 3	6. 5 / 3 .6. 5/2 _ _	jag h	C78	W100	U62	K67	30	60	22	−8	Nej		12{ 5/2 +10 {6} }
Dodeka- dodekaeder		2 | 5 5/2 _ _	( 5 / 2 , 5) 2	jag h	C45	W073	U36	K41	30	60	24	−6	Ja	3	12{5} 5/2 } +12 {
Stor icosihemidodekaeder		3 / 2 3 | 5/3 _ _	10/3 _ _ . 3/2 _ _ . 10/3 .3 _ _	jag h	C85	W106	U71	K76	30	60	26	−4	Nej		20{3} +6 { 10/3 }
Stor icosidodecahedron		2 | 3 5/2 _ _	( 5 / 2 .3) 2	jag h	C70	W094	U54	K59	30	60	32	2	Ja	7	20{3} +12 { 5/2 }
Cubitruncated cuboctahedron		4 / 3 3 4 |	8/3 _ _ .6.8	O h	C52	W079	U16	K21	48	72	20	−4	Ja	4	8/3 } +6 {8} +6{
Stor stympad cuboctahedron		4 / 3 2 3 |	_ 8/3 .4 . 6/5 _ _	O h	C67	W093	U20	K25	48	72	26	2	Ja	1	{4} 8/3 } +8 {6}+6{
Stympad stor dodekaeder		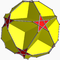2 5 / 2 | 5	10.10. 5/2 _ _	jag h	C47	W075	U37	K42	60	90	24	−6	Ja	3	12{ 5/2 +12 {10} }
Liten stjärnformad stympad dodekaeder		2 5 | 5/3 _ _	10/3 _ _ . 10/3 .5 _ _	jag h	C74	W097	U58	K63	60	90	24	−6	Ja	9	12{5} +12 { 10/3 }
Stor stjärnformad stympad dodekaeder		2 3 | 5/3 _ _	10/3 _ _ . 10/3 .3 _ _	jag h	C83	W104	U66	K71	60	90	32	2	Ja	13	20{3} +12 { 10/3 }
Stympad stor ikosaeder		2 5 / 2 | 3	6.6. 5/2 _ _	jag h	C71	W095	U55	K60	60	90	32	2	Ja	7	12{ 5/2 +20 {6} }
Stor dodecikosahedron		3 5 / 3 ( 3 / 2 5 / 2 ) |	6. 10/3 _ _ . 6/5 _ _ . 10/7 _ _	jag h	C79	W101	U63	K68	60	120	32	−28	Nej		20{6} +12 10/3 } {
Stor rhombidodecahedron		2 5 / 3 ( 3 / 2 5 / 4 ) |	4. 10/3 _ _ . 4/3 _ _ . 10/7 _ _	jag h	C89	W109	U73	K78	60	120	42	−18	Nej		30{4} +12 { 10/3 }
Icosidodeca- dodecahedron		5 / 3 5 | 3	6. 5 / 3 .6.5	jag h	C56	W083	U44	K49	60	120	44	−16	Ja	4	12{5}+12{ {6} 5/2 } +20
Liten ditrigonal dodecikosidodekaeder		5 / 3 3 | 5	10. 5 / 3 .10.3	jag h	C55	W082	U43	K48	60	120	44	−16	Ja	4	20{3}+12{ 5/2 +12 {10} }
Stor ditrigonal dodecikosidodekaeder		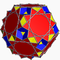3 5 | 5/3 _ _	_ 10/3 .3 . 10/3 .5 _ _	jag h	C54	W081	U42	K47	60	120	44	−16	Ja	4	20{3}+12{5} +12 10/3 } {
Stor dodecikosidodekaeder _		5 / 2 3 | 5/3 _ _	10/3 _ _ . 5/2 _ _ . 10/3 .3 _ _	jag h	C77	W099	U61	K66	60	120	44	−16	Ja	10	20{3} } } +12 { 10/3 { 5/2 +12
Liten icosikosidodekaeder		5 / 2 3 | 3	6. 5 / 2 .6.3	jag h	C40	W071	U31	K36	60	120	52	−8	Ja	2	20{3}+12{ {6} 5/2 } +20
Rhombidodeca- dodecahedron		5 / 2 5 | 2	4. 5 / 2 .4.5	jag h	C48	W076	U38	K43	60	120	54	−6	Ja	3	30{4}+12{5} +12 5/2 } {
Stor rhombicosi- dodecahedron		5 / 3 3 | 2	4. 5 / 3 .4.3	jag h	C84	W105	U67	K72	60	120	62	2	Ja	13	20{3}+30{4} +12 5/2 } {
Icositruncated dodeca- dodecahedron		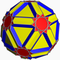3 5 5 / 3 |	10/3 .6.10 _ _	jag h	C57	W084	U45	K50	120	180	44	−16	Ja	4	20{6}+12{10} +12 { 10/3 }
Stympad dodeka- dodekaeder		2 5 5 / 3 |	_ 10/3 .4 . 10/9 _ _	jag h	C75	W098	U59	K64	120	180	54	−6	Ja	3	30{4}+12{10} +12 { 10/3 }
Stor stympad icosidodecahedron		2 3 5 / 3 |	10/3 .4.6 _ _	jag h	C87	W108	U68	K73	120	180	62	2	Ja	13	30{4}+20{6} +12 { 10/3 }
Snub dodeca- dodecahedron		| 2 5 / 2 5	3.3. 5/2 _ _ .3.5	jag	C49	W111	U40	K45	60	150	84	−6	Ja	3	60{3}+12{5} 5/2 } +12 {
Inverterad snubbig dodeka- dodekaeder		| _ 5/3 2 5	3. 5 / 3 .3.3.5	jag	C76	W114	U60	K65	60	150	84	−6	Ja	9	60{3}+12{5} +12 5/2 } {
Stor snubbig icosidodecahedron		| 2 5 / 2 3	3 4 . 5/2 _ _	jag	C73	W113	U57	K62	60	150	92	2	Ja	7	20 +60){3}+12 { 5/2 }
Stor inverterad snub icosidodecahedron		| _ 5/3 2 3	3 4 . 5/3 _ _	jag	C88	W116	U69	K74	60	150	92	2	Ja	13	20 +60){3}+12 { 5/2 }
Stor retrosnub icosidodecahedron		| 2 3 / 2 5 / 3	(3 4 . 5 / 2 )/2	jag	C90	W117	U74	K79	60	150	92	2	Ja	37	20 +60){3}+12 { 5/2 }
Stor snubbig dodecikosidodekaeder _		| 5/3 _ _ 5/2 _ _ 3	3 3 . _ 5/3 .3 . 5/2 _ _	jag	C80	W115	U64	K69	60	180	104	−16	Ja	10	5/2 } 60 ){3}+(12+12) {
Snub icosidodeca- dodecahedron		| _ 5/3 3 5	3 3 .5.3. 5/3 _ _	jag	C58	W112	U46	K51	60	180	104	−16	Ja	4	20 +60){3}+12{5}+12 { 5/2 }
Liten snubbig icos- icosidodecahedron		| _ 5/2 3 3	3 5 . 5/2 _ _	jag h	C41	W110	U32	K37	60	180	112	−8	Ja	2	40 +60){3}+12 { 5/2 }
Liten retrosnub icosicosidodecahedron		| 3/2 3/2 _ _ _ 5/2 _ _ _	(3 5 . 5 / 2 )/2	jag h	C91	W118	U72	K77	60	180	112	−8	Ja	38	40 +60){3}+12 { 5/2 }
Stor dirhombicosi- dodekaeder		| 3 / 2 5 / 3 3 5 / 2	(4. 5 / 3 .4.3. 4. 5 / 2 .4. 3 / 2 )/2	jag h	C92	W119	U75	K80	60	240	124	−56	Nej		5/2 {3} +60 {4}+24{ }

Specialfall

namn	Bild	Wyth sym	Vert. fikon	Sym.	C#	W#	U#	K#	Vert.	Kanter	Ansikten	Chi	Orienterbar ?	Dens.	Ansikten efter typ
Stor disnub dirhombidodecahedron		| ( 3 / 2 ) 5 / 3 (3) 5 / 2	( 5 / 2 .4.3.3.3.4. 5 / 3 . 4. 3 / 2 . 3 / 2 . 3 / 2 .4)/2	jag h	—	—	—	—	60	360 (*)	204	−96	Nej		120 {3} 5/2 } +60{4}+24{

Den stora disnub dirhombidodecahedron har 240 av sina 360 kanter som sammanfaller i rymden i 120 par. På grund av denna kantdegeneration anses den inte alltid vara en enhetlig polyeder.

Kolumn nyckel

• Enhetlig indexering: U01–U80 (Tetrahedron först, prismor vid 76+)
• Kaleido mjukvaruindexering: K01–K80 (K _n = U _{n –5} för n = 6 till 80) (prismor 1–5, Tetrahedron etc. 6+)
• Magnus Wenninger Polyhedron Modeller: W001-W119
  • 1–18: 5 konvexa regelbundna och 13 konvexa halvregelbundna
  • 20–22, 41: 4 icke-konvexa regelbundna
  • 19–66: Speciella 48 stellationer/föreningar (icke-regelbundna anges inte på denna lista)
  • 67–109: 43 icke-konvexa non-snub uniform
  • 110–119: 10 icke-konvexa snubbuniform
• Chi: Euler-karaktäristiken , χ . Enhetliga plattsättningar på planet motsvarar en torustopologi, med Euler som är karakteristisk för noll.
• Densitet: Densiteten (polytopen) representerar antalet lindningar av en polyeder runt dess centrum. Detta lämnas tomt för icke- orienterbara polyedrar och hemipolyedrar (polyedrar med ytor som går genom sina centra), för vilka densiteten inte är väldefinierad.
• Anmärkning om vertexfigurbilder:
  • De vita polygonlinjerna representerar polygonen "vertexfigur". De färgade ansiktena är inkluderade på vertexfigurbilderna hjälper till att se deras relationer. Vissa av de korsande ytorna är visuellt felaktigt ritade eftersom de inte är korrekt genomskärda visuellt för att visa vilka delar som ligger framför.

Se även

• Lista över enhetliga polyedrar efter vertexfigur
• Lista över enhetliga polyedrar av Wythoff symbol
• Lista över enhetliga polyedrar av Schwarz triangel

•      Coxeter, Harold Scott MacDonald ; Longuet-Higgins, MS; Miller, JCP (1954). "Uniform polyedra". Philosophical Transactions of the Royal Society of London. Serie A. Matematiska och fysiska vetenskaper . Royal Society. 246 (916): 401–450. Bibcode : 1954RSPTA.246..401C . doi : 10.1098/rsta.1954.0003 . ISSN 0080-4614 . JSTOR 91532 . MR 0062446 . S2CID 202575183 .
•      Skilling, J. (1975). "Den kompletta uppsättningen av enhetliga polyedrar". Philosophical Transactions of the Royal Society of London. Serie A. Matematiska och fysiska vetenskaper . 278 (1278): 111–135. Bibcode : 1975RSPTA.278..111S . doi : 10.1098/rsta.1975.0022 . ISSN 0080-4614 . JSTOR 74475 . MR 0365333 . S2CID 122634260 .
•   Sopov, SP (1970). "Ett bevis på fullständigheten på listan över elementära homogena polyedrar". Ukrainskiui Geometricheskiui Sbornik (8): 139–156. MR 0326550 .
•   Wenninger, Magnus (1974). Polyedermodeller . Cambridge University Press. ISBN 0-521-09859-9 .
•   Wenninger, Magnus (1983). Dubbla modeller . Cambridge University Press. ISBN 0-521-54325-8 .

externa länkar

• Stella: Polyhedron Navigator – Programvara som kan generera och skriva ut nät för alla enhetliga polyedrar. Används för att skapa de flesta bilder på denna sida.
• Pappersmodeller
• Uniform indexering: U1-U80, (Tetrahedron först)
  • Uniform Polyhedra (80), Paul Bourke
  • Weisstein, Eric W. "Uniform Polyhedron" . MathWorld .
  • http://www.mathconsult.ch/showroom/unipoly
    • Alla enhetliga polyedrar efter rotationsgrupp
  • https://web.archive.org/web/20171110075259/http://gratrix.net/polyhedra/uniform/summary/
  • http://www.it-c.dk/edu/documentation/mathworks/math/math/u/u034.htm
  • http://www.buddenbooks.com/jb/uniform/
• Kaleido Indexering: K1-K80 (Pentagonal prisma först)
  • https://www.math.technion.ac.il/~rl/kaleido
    • https://web.archive.org/web/20110927223146/http://www.math.technion.ac.il/~rl/docs /uniform.pdf Uniform Solution for Uniform Polyhedra
  • http://bulatov.org/polyhedra/uniform
  • http://www.orchidpalms.com/polyhedra/uniform/uniform.html
• Även
  • http://www.polyedergarten.de/polyhedrix/e_klintro.htm