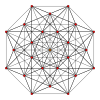
Uniform 7-polytop
I sjudimensionell geometri är en 7-polytop en polytop innehållen av 6-polytopfasetter. Varje 5-polytopås delas av exakt två 6-polytopa fasetter .
En enhetlig 7-polytop är en vars symmetrigrupp är transitiv på hörn och vars fasetter är enhetliga 6-polytoper .
Vanliga 7-polytoper
Vanliga 7-polytoper representeras av Schläfli-symbolen {p,q,r,s,t,u} med u {p,q,r,s,t} 6-polytoper facetter runt varje 4-yta.
Det finns exakt tre sådana konvexa vanliga 7-polytoper :
- {3,3,3,3,3,3} - 7-simplex
- {4,3,3,3,3,3} - 7-kub
- {3,3,3,3,3,4} - 7-ortoplex
Det finns inga icke-konvexa vanliga 7-polytoper.
Egenskaper
Topologin för en given 7-polytop definieras av dess Betti-tal och torsionskoefficienter .
Värdet av Euler-karaktäristiken som används för att karakterisera polyedrar generaliserar inte användbart till högre dimensioner, oavsett deras underliggande topologi. Denna otillräcklighet hos Euler-egenskapen för att tillförlitligt skilja mellan olika topologier i högre dimensioner ledde till upptäckten av de mer sofistikerade Betti-talen.
På liknande sätt är begreppet orienterbarhet för en polyeder otillräcklig för att karakterisera ytvridningarna av toroidformade polytoper, och detta ledde till användningen av torsionskoefficienter.
Enhetliga 7-polytoper av grundläggande Coxeter-grupper
Uniforma 7-polytoper med reflekterande symmetri kan genereras av dessa fyra Coxeter-grupper, representerade av permutationer av ringar i Coxeter- Dynkin-diagrammen :
| # | Coxeter grupp | Regelbundna och halvregelbundna former | Enhetligt antal | ||
|---|---|---|---|---|---|
| 1 | En 7 | [3 6 ] |
|
|
71 |
| 2 | B 7 | [4,3 5 ] |
|
|
127 + 32 |
| 3 | D 7 | [3 3,1,1 ] |
|
|
95 (0 unika) |
| 4 | E 7 | [3 3,2,1 ] |
|
127 | |
| Prismatiska ändliga Coxeter-grupper | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| # | Coxeter grupp | Coxeter diagram | |||||||||
| 6+1 | |||||||||||
| 1 | A 6 A 1 | [3 5 ]×[ ] |
|
||||||||
| 2 | BC 6 A 1 | [4,3 4 ]×[ ] |
|
||||||||
| 3 | D 6 A 1 | [3 3,1,1 ]×[ ] |
|
||||||||
| 4 | E 6 A 1 | [3 2,2,1 ]×[ ] |
|
||||||||
| 5+2 | |||||||||||
| 1 | A 5 I 2 (p) | [3,3,3]×[p] |
|
||||||||
| 2 | BC 5 I 2 (p) | [4,3,3]×[p] |
|
||||||||
| 3 | D 5 I 2 (p) | [3 2,1,1 ]×[p] |
|
||||||||
| 5+1+1 | |||||||||||
| 1 | A 5 A 1 2 | [3,3,3]×[ ] 2 |
|
||||||||
| 2 | BC 5 A 1 2 | [4,3,3]×[ ] 2 |
|
||||||||
| 3 | D 5 A 1 2 | [3 2,1,1 ]×[ ] 2 |
|
||||||||
| 4+3 | |||||||||||
| 1 | A 4 A 3 | [3,3,3]×[3,3] |
|
||||||||
| 2 | A 4 B 3 | [3,3,3]×[4,3] |
|
||||||||
| 3 | A 4 H 3 | [3,3,3]×[5,3] |
|
||||||||
| 4 | BC 4 A 3 | [4,3,3]×[3,3] |
|
||||||||
| 5 | BC 4 B 3 | [4,3,3]×[4,3] |
|
||||||||
| 6 | BC 4 H 3 | [4,3,3]×[5,3] |
|
||||||||
| 7 | H 4 A 3 | [5,3,3]×[3,3] |
|
||||||||
| 8 | H 4 B 3 | [5,3,3]×[4,3] |
|
||||||||
| 9 | H 4 H 3 | [5,3,3]×[5,3] |
|
||||||||
| 10 | F 4 A 3 | [3,4,3]×[3,3] |
|
||||||||
| 11 | F 4 B 3 | [3,4,3]×[4,3] |
|
||||||||
| 12 | F 4 H 3 | [3,4,3]×[5,3] |
|
||||||||
| 13 | D 4 A 3 | [3 1,1,1 ]×[3,3] |
|
||||||||
| 14 | D 4 B 3 | [3 1,1,1 ]×[4,3] |
|
||||||||
| 15 | D4H3 _ _ _ | [3 1,1,1 ]×[5,3] |
|
||||||||
| 4+2+1 | |||||||||||
| 1 | A 4 I 2 (p) A 1 | [3,3,3]×[p]×[ ] |
|
||||||||
| 2 | BC 4 I 2 (p)A 1 | [4,3,3]×[p]×[ ] |
|
||||||||
| 3 | F 4 I 2 (p)A 1 | [3,4,3]×[p]×[ ] |
|
||||||||
| 4 | H4I2 ( p ) A1 _ | [5,3,3]×[p]×[ ] |
|
||||||||
| 5 | D 4 I 2 (p) A 1 | [3 1,1,1 ]×[p]×[ ] |
|
||||||||
| 4+1+1+1 | |||||||||||
| 1 | A 4 A 1 3 | [3,3,3]×[ ] 3 |
|
||||||||
| 2 | BC 4 A 1 3 | [4,3,3]×[ ] 3 |
|
||||||||
| 3 | F 4 A 1 3 | [3,4,3]×[ ] 3 |
|
||||||||
| 4 | H 4 A 1 3 | [5,3,3]×[ ] 3 |
|
||||||||
| 5 | D 4 A 1 3 | [3 1,1,1 ]×[ ] 3 |
|
||||||||
| 3+3+1 | |||||||||||
| 1 | A 3 A 3 A 1 | [3,3]×[3,3]×[ ] |
|
||||||||
| 2 | A 3 B 3 A 1 | [3,3]×[4,3]×[ ] |
|
||||||||
| 3 | A 3 H 3 A 1 | [3,3]×[5,3]×[ ] |
|
||||||||
| 4 | BC 3 B 3 A 1 | [4,3]×[4,3]×[ ] |
|
||||||||
| 5 | BC 3 H 3 A 1 | [4,3]×[5,3]×[ ] |
|
||||||||
| 6 | H 3 A 3 A 1 | [5,3]×[5,3]×[ ] |
|
||||||||
| 3+2+2 | |||||||||||
| 1 | A 3 I 2 (p) I 2 (q) | [3,3]×[p]×[q] |
|
||||||||
| 2 | BC 3 I 2 (p) I 2 (q) | [4,3]×[p]×[q] |
|
||||||||
| 3 | H 3 I 2 (p) I 2 (q) | [5,3]×[p]×[q] |
|
||||||||
| 3+2+1+1 | |||||||||||
| 1 | A 3 I 2 (p) A 1 2 | [3,3]×[p]×[ ] 2 |
|
||||||||
| 2 | BC 3 I 2 (p) A 1 2 | [4,3]×[p]×[ ] 2 |
|
||||||||
| 3 | H3I2 ( p ) A12 _ _ | [5,3]×[p]×[ ] 2 |
|
||||||||
| 3+1+1+1+1 | |||||||||||
| 1 | A 3 A 1 4 | [3,3]×[ ] 4 |
|
||||||||
| 2 | BC 3 A 1 4 | [4,3]×[ ] 4 |
|
||||||||
| 3 | H 3 A 1 4 | [5,3]×[ ] 4 |
|
||||||||
| 2+2+2+1 | |||||||||||
| 1 | I2 (p)I2 ( q)I2 ( r ) A1 | [p]×[q]×[r]×[ ] |
|
||||||||
| 2+2+1+1+1 | |||||||||||
| 1 | I 2 (p) I 2 (q) A 1 3 | [p]×[q]×[ ] 3 |
|
||||||||
| 2+1+1+1+1+1 | |||||||||||
| 1 | I 2 (p)A 1 5 | [p]×[ ] 5 |
|
||||||||
| 1+1+1+1+1+1+1 | |||||||||||
| 1 | A 17 _ | [ ] 7 |
|
||||||||
Familjen A 7
A 7- familjen har symmetri av ordningen 40320 (8 factorial ).
Det finns 71 (64+8-1) former baserade på alla permutationer av Coxeter-Dynkin-diagrammen med en eller flera ringar. Alla 71 är uppräknade nedan. Norman Johnsons trunkeringsnamn anges. Bowers namn och akronym ges också för korsreferenser.
Se även en lista över A7-polytoper för symmetriska Coxeter- plangrafer för dessa polytoper.
| A 7 enhetliga polytoper | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| # | Coxeter-Dynkin diagram |
Trunkeringsindex _ |
Johnson namn Bowers namn (och akronym) |
Baspunkt | Element räknas | ||||||
| 6 | 5 | 4 | 3 | 2 | 1 | 0 | |||||
| 1 | t0 | 7-simplex (oca) | (0,0,0,0,0,0,0,1) | 8 | 28 | 56 | 70 | 56 | 28 | 8 | |
| 2 | t 1 | Rättad 7-simplex (roc) | (0,0,0,0,0,0,1,1) | 16 | 84 | 224 | 350 | 336 | 168 | 28 | |
| 3 | t 2 | Birektifierad 7-simplex (broc) | (0,0,0,0,0,1,1,1) | 16 | 112 | 392 | 770 | 840 | 420 | 56 | |
| 4 | t 3 | Trirectified 7-simplex (he) | (0,0,0,0,1,1,1,1) | 16 | 112 | 448 | 980 | 1120 | 560 | 70 | |
| 5 | t 0,1 | Trunkerad 7-simplex (toc) | (0,0,0,0,0,0,1,2) | 16 | 84 | 224 | 350 | 336 | 196 | 56 | |
| 6 | t 0,2 | Kantellerad 7-simplex (saro) | (0,0,0,0,0,1,1,2) | 44 | 308 | 980 | 1750 | 1876 | 1008 | 168 | |
| 7 | t 1,2 | Bitruncated 7-simplex (bittoc) | (0,0,0,0,0,1,2,2) | 588 | 168 | ||||||
| 8 | t 0,3 | Runcinerad 7-simplex (spo) | (0,0,0,0,1,1,1,2) | 100 | 756 | 2548 | 4830 | 4760 | 2100 | 280 | |
| 9 | t 1,3 | Bikantellerad 7-simplex (sabro) | (0,0,0,0,1,1,2,2) | 2520 | 420 | ||||||
| 10 | t 2,3 | Tritruncated 7-simplex (tattoc) | (0,0,0,0,1,2,2,2) | 980 | 280 | ||||||
| 11 | t 0,4 | Stericated 7-simplex (sco) | (0,0,0,1,1,1,1,2) | 2240 | 280 | ||||||
| 12 | t 1,4 | Biruncinerad 7-simplex (sibpo) | (0,0,0,1,1,1,2,2) | 4200 | 560 | ||||||
| 13 | t 2,4 | Trikantellerad 7-simplex (stiroh) | (0,0,0,1,1,2,2,2) | 3360 | 560 | ||||||
| 14 | t 0,5 | Pentellated 7-simplex (seto) | (0,0,1,1,1,1,1,2) | 1260 | 168 | ||||||
| 15 | t 1,5 | Bistericated 7-simplex (sabach) | (0,0,1,1,1,1,2,2) | 3360 | 420 | ||||||
| 16 | t 0,6 | Hexicerad 7-simplex (suph) | (0,1,1,1,1,1,1,2) | 336 | 56 | ||||||
| 17 | t 0,1,2 | Cantitruncated 7-simplex (garo) | (0,0,0,0,0,1,2,3) | 1176 | 336 | ||||||
| 18 | t 0,1,3 | Runcitruncated 7-simplex (patto) | (0,0,0,0,1,1,2,3) | 4620 | 840 | ||||||
| 19 | t 0,2,3 | Runcicantellated 7-simplex (paro) | (0,0,0,0,1,2,2,3) | 3360 | 840 | ||||||
| 20 | t 1,2,3 | Bicantitruncated 7-simplex (gabro) | (0,0,0,0,1,2,3,3) | 2940 | 840 | ||||||
| 21 | t 0,1,4 | Steritruncated 7-simplex (cato) | (0,0,0,1,1,1,2,3) | 7280 | 1120 | ||||||
| 22 | t 0,2,4 | Steriskantellerad 7-simplex (caro) | (0,0,0,1,1,2,2,3) | 10080 | 1680 | ||||||
| 23 | t 1,2,4 | Biruncitruncated 7-simplex (bipto) | (0,0,0,1,1,2,3,3) | 8400 | 1680 | ||||||
| 24 | t 0,3,4 | Steriruncinerad 7-simplex (cepo) | (0,0,0,1,2,2,2,3) | 5040 | 1120 | ||||||
| 25 | t 1,3,4 | Biruncicantellated 7-simplex (bipro) | (0,0,0,1,2,2,3,3) | 7560 | 1680 | ||||||
| 26 | t 2,3,4 | Trikantitrunkerad 7-simplex (gatroh) | (0,0,0,1,2,3,3,3) | 3920 | 1120 | ||||||
| 27 | t 0,1,5 | Pentitruncated 7-simplex (teto) | (0,0,1,1,1,1,2,3) | 5460 | 840 | ||||||
| 28 | t 0,2,5 | Penticantellated 7-simplex (tero) | (0,0,1,1,1,2,2,3) | 11760 | 1680 | ||||||
| 29 | t 1,2,5 | Bisteritruncated 7-simplex (bacto) | (0,0,1,1,1,2,3,3) | 9240 | 1680 | ||||||
| 30 | t 0,3,5 | Pentiruncinerad 7-simplex (tepo) | (0,0,1,1,2,2,2,3) | 10920 | 1680 | ||||||
| 31 | t 1,3,5 | Bistericantellated 7-simplex (bacroh) | (0,0,1,1,2,2,3,3) | 15120 | 2520 | ||||||
| 32 | t 0,4,5 | Pentistericerad 7-simplex (teco) | (0,0,1,2,2,2,2,3) | 4200 | 840 | ||||||
| 33 | t 0,1,6 | Hexitruncated 7-simplex (puto) | (0,1,1,1,1,1,2,3) | 1848 | 336 | ||||||
| 34 | t 0,2,6 | Hexicantellated 7-simplex (puro) | (0,1,1,1,1,2,2,3) | 5880 | 840 | ||||||
| 35 | t 0,3,6 | Hexiruncinerad 7-simplex (puph) | (0,1,1,1,2,2,2,3) | 8400 | 1120 | ||||||
| 36 | t 0,1,2,3 | Runcicantitruncated 7-simplex (gapo) | (0,0,0,0,1,2,3,4) | 5880 | 1680 | ||||||
| 37 | t 0,1,2,4 | Stericantitruncated 7-simplex (cagro) | (0,0,0,1,1,2,3,4) | 16800 | 3360 | ||||||
| 38 | t 0,1,3,4 | Sterirruncated 7-simplex (capto) | (0,0,0,1,2,2,3,4) | 13440 | 3360 | ||||||
| 39 | t 0,2,3,4 | Steriruncikantellerad 7-simplex (capro) | (0,0,0,1,2,3,3,4) | 13440 | 3360 | ||||||
| 40 | t 1,2,3,4 | Biruncicantitruncated 7-simplex (gibpo) | (0,0,0,1,2,3,4,4) | 11760 | 3360 | ||||||
| 41 | t 0,1,2,5 | Penticantitruncated 7-simplex (tegro) | (0,0,1,1,1,2,3,4) | 18480 | 3360 | ||||||
| 42 | t 0,1,3,5 | Pentiruncitruncated 7-simplex (tapto) | (0,0,1,1,2,2,3,4) | 27720 | 5040 | ||||||
| 43 | t 0,2,3,5 | Pentiruncicantellated 7-simplex (tapro) | (0,0,1,1,2,3,3,4) | 25200 | 5040 | ||||||
| 44 | t 1,2,3,5 | Bistericantitruncated 7-simplex (bacogro) | (0,0,1,1,2,3,4,4) | 22680 | 5040 | ||||||
| 45 | t 0,1,4,5 | Pentisteritruncated 7-simplex (tecto) | (0,0,1,2,2,2,3,4) | 15120 | 3360 | ||||||
| 46 | t 0,2,4,5 | Pentistericantellated 7-simplex (tecro) | (0,0,1,2,2,3,3,4) | 25200 | 5040 | ||||||
| 47 | t 1,2,4,5 | Bisteriruncitruncated 7-simplex (bicpath) | (0,0,1,2,2,3,4,4) | 20160 | 5040 | ||||||
| 48 | t 0,3,4,5 | Pentisteriruncinerad 7-simplex (tacpo) | (0,0,1,2,3,3,3,4) | 15120 | 3360 | ||||||
| 49 | t 0,1,2,6 | Hexicantitruncated 7-simplex (pugro) | (0,1,1,1,1,2,3,4) | 8400 | 1680 | ||||||
| 50 | t 0,1,3,6 | Hexiruncated 7-simplex (pugato) | (0,1,1,1,2,2,3,4) | 20160 | 3360 | ||||||
| 51 | t 0,2,3,6 | Hexiruncicantellated 7-simplex (pugro) | (0,1,1,1,2,3,3,4) | 16800 | 3360 | ||||||
| 52 | t 0,1,4,6 | Hexisteritruncated 7-simplex (pucto) | (0,1,1,2,2,2,3,4) | 20160 | 3360 | ||||||
| 53 | t 0,2,4,6 | Hexistericantellated 7-simplex (pucroh) | (0,1,1,2,2,3,3,4) | 30240 | 5040 | ||||||
| 54 | t 0,1,5,6 | Hexipentitruncated 7-simplex (putath) | (0,1,2,2,2,2,3,4) | 8400 | 1680 | ||||||
| 55 | t 0,1,2,3,4 | Steriruncicantitruncated 7-simplex (gecco) | (0,0,0,1,2,3,4,5) | 23520 | 6720 | ||||||
| 56 | t 0,1,2,3,5 | Pentiruncicanantitruncated 7-simplex (tegapo) | (0,0,1,1,2,3,4,5) | 45360 | 10080 | ||||||
| 57 | t 0,1,2,4,5 | Pentistericantitruncated 7-simplex (tecagro) | (0,0,1,2,2,3,4,5) | 40320 | 10080 | ||||||
| 58 | t 0,1,3,4,5 | Pentisteriruncitruncated 7-simplex (tacpeto) | (0,0,1,2,3,3,4,5) | 40320 | 10080 | ||||||
| 59 | t 0,2,3,4,5 | Pentisteriruncicantellated 7-simplex (tacpro) | (0,0,1,2,3,4,4,5) | 40320 | 10080 | ||||||
| 60 | t 1,2,3,4,5 | Bisteriruncicanantitruncated 7-simplex (gabach) | (0,0,1,2,3,4,5,5) | 35280 | 10080 | ||||||
| 61 | t 0,1,2,3,6 | Hexiruncicantitruncated 7-simplex (pugopo) | (0,1,1,1,2,3,4,5) | 30240 | 6720 | ||||||
| 62 | t 0,1,2,4,6 | Hexistericantitruncated 7-simplex (pucagro) | (0,1,1,2,2,3,4,5) | 50400 | 10080 | ||||||
| 63 | t 0,1,3,4,6 | Hexisteriuncitruncated 7-simplex (pucpato) | (0,1,1,2,3,3,4,5) | 45360 | 10080 | ||||||
| 64 | t 0,2,3,4,6 | Hexisteriruncicantellated 7-simplex (pucproh) | (0,1,1,2,3,4,4,5) | 45360 | 10080 | ||||||
| 65 | t 0,1,2,5,6 | Hexipenticantitruncated 7-simplex (putagro) | (0,1,2,2,2,3,4,5) | 30240 | 6720 | ||||||
| 66 | t 0,1,3,5,6 | Hexipentiruncitruncated 7-simplex (putpath) | (0,1,2,2,3,3,4,5) | 50400 | 10080 | ||||||
| 67 | t 0,1,2,3,4,5 | Pentisteriruncicanantitruncated 7-simplex (geto) | (0,0,1,2,3,4,5,6) | 70560 | 20160 | ||||||
| 68 | t 0,1,2,3,4,6 | Hexisteriruncicanantitruncated 7-simplex (pugaco) | (0,1,1,2,3,4,5,6) | 80640 | 20160 | ||||||
| 69 | t 0,1,2,3,5,6 | Hexipentiruncicanantitruncated 7-simplex (putgapo) | (0,1,2,2,3,4,5,6) | 80640 | 20160 | ||||||
| 70 | t 0,1,2,4,5,6 | Hexipentistericantitruncated 7-simplex (putcagroh) | (0,1,2,3,3,4,5,6) | 80640 | 20160 | ||||||
| 71 | t 0,1,2,3,4,5,6 | Omnitruncerad 7-simplex (guph) | (0,1,2,3,4,5,6,7) | 141120 | 40320 | ||||||
Familjen B 7
B 7 -familjen har symmetri av ordningen 645120 (7 factorial x 2 7 ).
Det finns 127 former baserade på alla permutationer av Coxeter-Dynkin-diagrammen med en eller flera ringar. Johnson och Bowers namn.
Se även en lista över B7-polytoper för symmetriska Coxeter- plangrafer för dessa polytoper.
| B 7 enhetliga polytoper | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| # |
Coxeter-Dynkin diagram t-notation |
Namn (BSA) | Baspunkt | Element räknas | |||||||
| 6 | 5 | 4 | 3 | 2 | 1 | 0 | |||||
| 1 |
0 t {3,3,3,3,3,4} |
7-ortoplex (zee) | (0,0,0,0,0,0,1)√2 | 128 | 448 | 672 | 560 | 280 | 84 | 14 | |
| 2 |
t 1 {3,3,3,3,3,4} |
Rectified 7-ortoplex (rez) | (0,0,0,0,0,1,1)√2 | 142 | 1344 | 3360 | 3920 | 2520 | 840 | 84 | |
| 3 |
t 2 {3,3,3,3,3,4} |
Birectified 7-ortoplex (barz) | (0,0,0,0,1,1,1)√2 | 142 | 1428 | 6048 | 10640 | 8960 | 3360 | 280 | |
| 4 |
t 3 {4,3,3,3,3,3} |
Trekorrigerad 7-kub (sez) | (0,0,0,1,1,1,1)√2 | 142 | 1428 | 6328 | 14560 | 15680 | 6720 | 560 | |
| 5 |
t 2 {4,3,3,3,3,3} |
Birektifierad 7-kub (bersa) | (0,0,1,1,1,1,1)√2 | 142 | 1428 | 5656 | 11760 | 13440 | 6720 | 672 | |
| 6 |
t 1 {4,3,3,3,3,3} |
Rättad 7-kub (rasa) | (0,1,1,1,1,1,1)√2 | 142 | 980 | 2968 | 5040 | 5152 | 2688 | 448 | |
| 7 |
0 t {4,3,3,3,3,3} |
7-kub (hept) | (0,0,0,0,0,0,0)√2 + (1,1,1,1,1,1,1) | 14 | 84 | 280 | 560 | 672 | 448 | 128 | |
| 8 |
t 0,1 {3,3,3,3,3,4} |
Trunkerad 7-ortoplex (Taz) | (0,0,0,0,0,1,2)√2 | 142 | 1344 | 3360 | 4760 | 2520 | 924 | 168 | |
| 9 |
t 0,2 {3,3,3,3,3,4} |
Kantellerad 7-ortoplex (Sarz) | (0,0,0,0,1,1,2)√2 | 226 | 4200 | 15456 | 24080 | 19320 | 7560 | 840 | |
| 10 |
t 1,2 {3,3,3,3,3,4} |
Bitruncated 7-ortoplex (Botaz) | (0,0,0,0,1,2,2)√2 | 4200 | 840 | ||||||
| 11 |
t 0,3 {3,3,3,3,3,4} |
Runcinerad 7-ortoplex (Spaz) | (0,0,0,1,1,1,2)√2 | 23520 | 2240 | ||||||
| 12 |
t 1,3 {3,3,3,3,3,4} |
Bikantellerad 7-ortoplex (Sebraz) | (0,0,0,1,1,2,2)√2 | 26880 | 3360 | ||||||
| 13 |
t 2,3 {3,3,3,3,3,4} |
Tritrunkerad 7-ortoplex (Totaz) | (0,0,0,1,2,2,2)√2 | 10080 | 2240 | ||||||
| 14 |
t 0,4 {3,3,3,3,3,4} |
Stericated 7-ortoplex (Scaz) | (0,0,1,1,1,1,2)√2 | 33600 | 3360 | ||||||
| 15 |
t 1,4 {3,3,3,3,3,4} |
Biruncinerad 7-ortoplex (Sibpaz) | (0,0,1,1,1,2,2)√2 | 60480 | 6720 | ||||||
| 16 |
t 2,4 {4,3,3,3,3,3} |
Trikantellerad 7-kub (Strasaz) | (0,0,1,1,2,2,2)√2 | 47040 | 6720 | ||||||
| 17 |
t 2,3 {4,3,3,3,3,3} |
Tritrunkerad 7-kub (Tatsa) | (0,0,1,2,2,2,2)√2 | 13440 | 3360 | ||||||
| 18 |
t 0,5 {3,3,3,3,3,4} |
Pentellerad 7-ortoplex (Staz) | (0,1,1,1,1,1,2)√2 | 20160 | 2688 | ||||||
| 19 |
t 1,5 {4,3,3,3,3,3} |
Bistericated 7-kub (Sabcosaz) | (0,1,1,1,1,2,2)√2 | 53760 | 6720 | ||||||
| 20 |
t 1,4 {4,3,3,3,3,3} |
Biruncinerad 7-kub (Sibposa) | (0,1,1,1,2,2,2)√2 | 67200 | 8960 | ||||||
| 21 |
t 1,3 {4,3,3,3,3,3} |
Bikantellerad 7-kub (Sibrosa) | (0,1,1,2,2,2,2)√2 | 40320 | 6720 | ||||||
| 22 |
t 1,2 {4,3,3,3,3,3} |
Bitrunkerad 7-kub (Betsa) | (0,1,2,2,2,2,2)√2 | 9408 | 2688 | ||||||
| 23 |
t 0,6 {4,3,3,3,3,3} |
Hexicerad 7-kub (Supposaz) | (0,0,0,0,0,0,1)√2 + (1,1,1,1,1,1,1) | 5376 | 896 | ||||||
| 24 |
t 0,5 {4,3,3,3,3,3} |
Pentellerad 7-kub (Stesa) | (0,0,0,0,0,1,1)√2 + (1,1,1,1,1,1,1) | 20160 | 2688 | ||||||
| 25 |
t 0,4 {4,3,3,3,3,3} |
Stericerad 7-kub (Scosa) | (0,0,0,0,1,1,1)√2 + (1,1,1,1,1,1,1) | 35840 | 4480 | ||||||
| 26 |
t 0,3 {4,3,3,3,3,3} |
Runcinerad 7-kub (Spesa) | (0,0,0,1,1,1,1)√2 + (1,1,1,1,1,1,1) | 33600 | 4480 | ||||||
| 27 |
t 0,2 {4,3,3,3,3,3} |
Kantellerad 7-kub (Sersa) | (0,0,1,1,1,1,1)√2 + (1,1,1,1,1,1,1) | 16128 | 2688 | ||||||
| 28 |
t 0,1 {4,3,3,3,3,3} |
Trunkerad 7-kub (Tasa) | (0,1,1,1,1,1,1)√2 + (1,1,1,1,1,1,1) | 142 | 980 | 2968 | 5040 | 5152 | 3136 | 896 | |
| 29 |
t 0,1,2 {3,3,3,3,3,4} |
Cantitruncated 7-ortoplex (Garz) | (0,1,2,3,3,3,3)√2 | 8400 | 1680 | ||||||
| 30 |
t 0,1,3 {3,3,3,3,3,4} |
Runcitruncated 7-ortoplex (Potaz) | (0,1,2,2,3,3,3)√2 | 50400 | 6720 | ||||||
| 31 |
t 0,2,3 {3,3,3,3,3,4} |
Runcikantellerad 7-ortoplex (Parz) | (0,1,1,2,3,3,3)√2 | 33600 | 6720 | ||||||
| 32 |
t 1,2,3 {3,3,3,3,3,4} |
Bicantitruncated 7-ortoplex (Gebraz) | (0,0,1,2,3,3,3)√2 | 30240 | 6720 | ||||||
| 33 |
t 0,1,4 {3,3,3,3,3,4} |
Steritruncated 7-ortoplex (Catz) | (0,0,1,1,1,2,3)√2 | 107520 | 13440 | ||||||
| 34 |
t 0,2,4 {3,3,3,3,3,4} |
Steriskantellerad 7-ortoplex (Craze) | (0,0,1,1,2,2,3)√2 | 141120 | 20160 | ||||||
| 35 |
t 1,2,4 {3,3,3,3,3,4} |
Biruncitruncated 7-ortoplex (döpa) | (0,0,1,1,2,3,3)√2 | 120960 | 20160 | ||||||
| 36 |
t 0,3,4 {3,3,3,3,3,4} |
Steriruncinerad 7-ortoplex (Copaz) | (0,1,1,1,2,3,3)√2 | 67200 | 13440 | ||||||
| 37 |
t 1,3,4 {3,3,3,3,3,4} |
Biruncicantellated 7-ortoplex (Boparz) | (0,0,1,2,2,3,3)√2 | 100800 | 20160 | ||||||
| 38 |
t 2,3,4 {4,3,3,3,3,3} |
Trikantitrunkerad 7-kub (Gotrasaz) | (0,0,0,1,2,3,3)√2 | 53760 | 13440 | ||||||
| 39 |
t 0,1,5 {3,3,3,3,3,4} |
Pentitruncated 7-ortoplex (Tetaz) | (0,1,1,1,1,2,3)√2 | 87360 | 13440 | ||||||
| 40 |
t 0,2,5 {3,3,3,3,3,4} |
Penticantellated 7-ortoplex (Teroz) | (0,1,1,1,2,2,3)√2 | 188160 | 26880 | ||||||
| 41 |
t 1,2,5 {3,3,3,3,3,4} |
Bisteritruncated 7-ortoplex (Boctaz) | (0,1,1,1,2,3,3)√2 | 147840 | 26880 | ||||||
| 42 |
t 0,3,5 {3,3,3,3,3,4} |
Pentiruncinerad 7-ortoplex (Topaz) | (0,1,1,2,2,2,3)√2 | 174720 | 26880 | ||||||
| 43 |
t 1,3,5 {4,3,3,3,3,3} |
Bisterikantellerad 7-kub (Bacresaz) | (0,1,1,2,2,3,3)√2 | 241920 | 40320 | ||||||
| 44 |
t 1,3,4 {4,3,3,3,3,3} |
Biruncicantellated 7-kub (Bopresa) | (0,1,1,2,3,3,3)√2 | 120960 | 26880 | ||||||
| 45 |
t 0,4,5 {3,3,3,3,3,4} |
Pentistericerad 7-ortoplex (Tocaz) | (0,1,2,2,2,2,3)√2 | 67200 | 13440 | ||||||
| 46 |
t 1,2,5 {4,3,3,3,3,3} |
Bisteritrunkerad 7-kub (Bactasa) | (0,1,2,2,2,3,3)√2 | 147840 | 26880 | ||||||
| 47 |
t 1,2,4 {4,3,3,3,3,3} |
Biruncitruncated 7-kub (Biptesa) | (0,1,2,2,3,3,3)√2 | 134400 | 26880 | ||||||
| 48 |
t 1,2,3 {4,3,3,3,3,3} |
Bicantitruncated 7-kub (Gibrosa) | (0,1,2,3,3,3,3)√2 | 47040 | 13440 | ||||||
| 49 |
t 0,1,6 {3,3,3,3,3,4} |
Hexitrunkerad 7-ortoplex (Putaz) | (0,0,0,0,0,1,2)√2 + (1,1,1,1,1,1,1) | 29568 | 5376 | ||||||
| 50 |
t 0,2,6 {3,3,3,3,3,4} |
Hexicantellated 7-ortoplex (Puraz) | (0,0,0,0,1,1,2)√2 + (1,1,1,1,1,1,1) | 94080 | 13440 | ||||||
| 51 |
t 0,4,5 {4,3,3,3,3,3} |
Pentistericerad 7-kub (Tacosa) | (0,0,0,0,1,2,2)√2 + (1,1,1,1,1,1,1) | 67200 | 13440 | ||||||
| 52 |
t 0,3,6 {4,3,3,3,3,3} |
Hexiruncinerad 7-kub (Pupsez) | (0,0,0,1,1,1,2)√2 + (1,1,1,1,1,1,1) | 134400 | 17920 | ||||||
| 53 |
t 0,3,5 {4,3,3,3,3,3} |
Pentiruncinerad 7-kub (Tapsa) | (0,0,0,1,1,2,2)√2 + (1,1,1,1,1,1,1) | 174720 | 26880 | ||||||
| 54 |
t 0,3,4 {4,3,3,3,3,3} |
Steriruncinerad 7-kub (Capsa) | (0,0,0,1,2,2,2)√2 + (1,1,1,1,1,1,1) | 80640 | 17920 | ||||||
| 55 |
t 0,2,6 {4,3,3,3,3,3} |
Hexicantellerad 7-kub (Purosa) | (0,0,1,1,1,1,2)√2 + (1,1,1,1,1,1,1) | 94080 | 13440 | ||||||
| 56 |
t 0,2,5 {4,3,3,3,3,3} |
Penticantellated 7-kub (Tersa) | (0,0,1,1,1,2,2)√2 + (1,1,1,1,1,1,1) | 188160 | 26880 | ||||||
| 57 |
t 0,2,4 {4,3,3,3,3,3} |
Steriskantellerad 7-kub (Carsa) | (0,0,1,1,2,2,2)√2 + (1,1,1,1,1,1,1) | 161280 | 26880 | ||||||
| 58 |
t 0,2,3 {4,3,3,3,3,3} |
Runcicantellated 7-kub (Parsa) | (0,0,1,2,2,2,2)√2 + (1,1,1,1,1,1,1) | 53760 | 13440 | ||||||
| 59 |
t 0,1,6 {4,3,3,3,3,3} |
Hexitrunkerad 7-kub (Putsa) | (0,1,1,1,1,1,2)√2 + (1,1,1,1,1,1,1) | 29568 | 5376 | ||||||
| 60 |
t 0,1,5 {4,3,3,3,3,3} |
Pentitruncated 7-kub (Tetsa) | (0,1,1,1,1,2,2)√2 + (1,1,1,1,1,1,1) | 87360 | 13440 | ||||||
| 61 |
t 0,1,4 {4,3,3,3,3,3} |
Steritruncated 7-kub (Catsa) | (0,1,1,1,2,2,2)√2 + (1,1,1,1,1,1,1) | 116480 | 17920 | ||||||
| 62 |
t 0,1,3 {4,3,3,3,3,3} |
Runcitruncated 7-kub (Petsa) | (0,1,1,2,2,2,2)√2 + (1,1,1,1,1,1,1) | 73920 | 13440 | ||||||
| 63 |
t 0,1,2 {4,3,3,3,3,3} |
Cantitruncated 7-kub (Gersa) | (0,1,2,2,2,2,2)√2 + (1,1,1,1,1,1,1) | 18816 | 5376 | ||||||
| 64 |
t 0,1,2,3 {3,3,3,3,3,4} |
Runcicantitruncated 7-ortoplex (Gopaz) | (0,1,2,3,4,4,4)√2 | 60480 | 13440 | ||||||
| 65 |
t 0,1,2,4 {3,3,3,3,3,4} |
Stericantitruncated 7-ortoplex (Cogarz) | (0,0,1,1,2,3,4)√2 | 241920 | 40320 | ||||||
| 66 |
t 0,1,3,4 {3,3,3,3,3,4} |
Steriruncated 7-ortoplex (Captaz) | (0,0,1,2,2,3,4)√2 | 181440 | 40320 | ||||||
| 67 |
t 0,2,3,4 {3,3,3,3,3,4} |
Steriruncikantellerad 7-ortoplex (Caparz) | (0,0,1,2,3,3,4)√2 | 181440 | 40320 | ||||||
| 68 |
t 1,2,3,4 {3,3,3,3,3,4} |
Biruncicantitruncated 7-ortoplex (Gibpaz) | (0,0,1,2,3,4,4)√2 | 161280 | 40320 | ||||||
| 69 |
t 0,1,2,5 {3,3,3,3,3,4} |
Penticantitruncated 7-ortoplex (Tograz) | (0,1,1,1,2,3,4)√2 | 295680 | 53760 | ||||||
| 70 |
t 0,1,3,5 {3,3,3,3,3,4} |
Pentiruncitruncated 7-ortoplex (Toptaz) | (0,1,1,2,2,3,4)√2 | 443520 | 80640 | ||||||
| 71 |
t 0,2,3,5 {3,3,3,3,3,4} |
Pentiruncicantellated 7-ortoplex (Toparz) | (0,1,1,2,3,3,4)√2 | 403200 | 80640 | ||||||
| 72 |
t 1,2,3,5 {3,3,3,3,3,4} |
Bistericantitruncated 7-ortoplex (Becogarz) | (0,1,1,2,3,4,4)√2 | 362880 | 80640 | ||||||
| 73 |
t 0,1,4,5 {3,3,3,3,3,4} |
Pentisteritruncated 7-ortoplex (Tacotaz) | (0,1,2,2,2,3,4)√2 | 241920 | 53760 | ||||||
| 74 |
t 0,2,4,5 {3,3,3,3,3,4} |
Pentistericantellated 7-ortoplex (Tocarz) | (0,1,2,2,3,3,4)√2 | 403200 | 80640 | ||||||
| 75 |
t 1,2,4,5 {4,3,3,3,3,3} |
Bisteriruncitruncated 7-kub (Bocaptosaz) | (0,1,2,2,3,4,4)√2 | 322560 | 80640 | ||||||
| 76 |
t 0,3,4,5 {3,3,3,3,3,4} |
Pentisteriruncinerad 7-ortoplex (Tecpaz) | (0,1,2,3,3,3,4)√2 | 241920 | 53760 | ||||||
| 77 |
t 1,2,3,5 {4,3,3,3,3,3} |
Bistericantitruncated 7-cube (Becgresa) | (0,1,2,3,3,4,4)√2 | 362880 | 80640 | ||||||
| 78 |
t 1,2,3,4 {4,3,3,3,3,3} |
Biruncicantitruncated 7-cube (Gibposa) | (0,1,2,3,4,4,4)√2 | 188160 | 53760 | ||||||
| 79 |
t 0,1,2,6 {3,3,3,3,3,4} |
Hexicantitruncated 7-ortoplex (Pugarez) | (0,0,0,0,1,2,3)√2 + (1,1,1,1,1,1,1) | 134400 | 26880 | ||||||
| 80 |
t 0,1,3,6 {3,3,3,3,3,4} |
Hexiruncated 7-ortoplex (Papataz) | (0,0,0,1,1,2,3)√2 + (1,1,1,1,1,1,1) | 322560 | 53760 | ||||||
| 81 |
t 0,2,3,6 {3,3,3,3,3,4} |
Hexiruncicantellated 7-ortoplex (Puparez) | (0,0,0,1,2,2,3)√2 + (1,1,1,1,1,1,1) | 268800 | 53760 | ||||||
| 82 |
t 0,3,4,5 {4,3,3,3,3,3} |
Pentisteriruncinerad 7-kub (Tecpasa) | (0,0,0,1,2,3,3)√2 + (1,1,1,1,1,1,1) | 241920 | 53760 | ||||||
| 83 |
t 0,1,4,6 {3,3,3,3,3,4} |
Hexisteritruncated 7-ortoplex (Pucotaz) | (0,0,1,1,1,2,3)√2 + (1,1,1,1,1,1,1) | 322560 | 53760 | ||||||
| 84 |
t 0,2,4,6 {4,3,3,3,3,3} |
Hexisterikantellerad 7-kub (Pucrosaz) | (0,0,1,1,2,2,3)√2 + (1,1,1,1,1,1,1) | 483840 | 80640 | ||||||
| 85 |
t 0,2,4,5 {4,3,3,3,3,3} |
Pentistericantellated 7-kub (Tecresa) | (0,0,1,1,2,3,3)√2 + (1,1,1,1,1,1,1) | 403200 | 80640 | ||||||
| 86 |
t 0,2,3,6 {4,3,3,3,3,3} |
Hexiruncicantellated 7-kub (Pupresa) | (0,0,1,2,2,2,3)√2 + (1,1,1,1,1,1,1) | 268800 | 53760 | ||||||
| 87 |
t 0,2,3,5 {4,3,3,3,3,3} |
Pentiruncicantellated 7-kub (Topresa) | (0,0,1,2,2,3,3)√2 + (1,1,1,1,1,1,1) | 403200 | 80640 | ||||||
| 88 |
t 0,2,3,4 {4,3,3,3,3,3} |
Steriruncikantellerad 7-kub (Copresa) | (0,0,1,2,3,3,3)√2 + (1,1,1,1,1,1,1) | 215040 | 53760 | ||||||
| 89 |
t 0,1,5,6 {4,3,3,3,3,3} |
Hexipentitrunkerad 7-kub (Putatosez) | (0,1,1,1,1,2,3)√2 + (1,1,1,1,1,1,1) | 134400 | 26880 | ||||||
| 90 |
t 0,1,4,6 {4,3,3,3,3,3} |
Hexisteritrunkerad 7-kub (Pacutsa) | (0,1,1,1,2,2,3)√2 + (1,1,1,1,1,1,1) | 322560 | 53760 | ||||||
| 91 |
t 0,1,4,5 {4,3,3,3,3,3} |
Pentisteritrunkerad 7-kub (Tecatsa) | (0,1,1,1,2,3,3)√2 + (1,1,1,1,1,1,1) | 241920 | 53760 | ||||||
| 92 |
t 0,1,3,6 {4,3,3,3,3,3} |
Hexiruncitruncated 7-kub (Pupetsa) | (0,1,1,2,2,2,3)√2 + (1,1,1,1,1,1,1) | 322560 | 53760 | ||||||
| 93 |
t 0,1,3,5 {4,3,3,3,3,3} |
Pentiruncitruncated 7-kub (Toptosa) | (0,1,1,2,2,3,3)√2 + (1,1,1,1,1,1,1) | 443520 | 80640 | ||||||
| 94 |
t 0,1,3,4 {4,3,3,3,3,3} |
Sterirruncated 7-kub (Captesa) | (0,1,1,2,3,3,3)√2 + (1,1,1,1,1,1,1) | 215040 | 53760 | ||||||
| 95 |
t 0,1,2,6 {4,3,3,3,3,3} |
Hexicantitruncated 7-cube (Pugrosa) | (0,1,2,2,2,2,3)√2 + (1,1,1,1,1,1,1) | 134400 | 26880 | ||||||
| 96 |
t 0,1,2,5 {4,3,3,3,3,3} |
Penticantitruncated 7-cube (Togresa) | (0,1,2,2,2,3,3)√2 + (1,1,1,1,1,1,1) | 295680 | 53760 | ||||||
| 97 |
t 0,1,2,4 {4,3,3,3,3,3} |
Stericantitruncated 7-kub (Cogarsa) | (0,1,2,2,3,3,3)√2 + (1,1,1,1,1,1,1) | 268800 | 53760 | ||||||
| 98 |
t 0,1,2,3 {4,3,3,3,3,3} |
Runcicantitruncated 7-cube (Gapsa) | (0,1,2,3,3,3,3)√2 + (1,1,1,1,1,1,1) | 94080 | 26880 | ||||||
| 99 |
t 0,1,2,3,4 {3,3,3,3,3,4} |
Steriruncicantitruncated 7-ortoplex (Gocaz) | (0,0,1,2,3,4,5)√2 | 322560 | 80640 | ||||||
| 100 |
t 0,1,2,3,5 {3,3,3,3,3,4} |
Pentiruncicanantitruncated 7-ortoplex (Tegopaz) | (0,1,1,2,3,4,5)√2 | 725760 | 161280 | ||||||
| 101 |
t 0,1,2,4,5 {3,3,3,3,3,4} |
Pentistericantitruncated 7-ortoplex (Tecagraz) | (0,1,2,2,3,4,5)√2 | 645120 | 161280 | ||||||
| 102 |
t 0,1,3,4,5 {3,3,3,3,3,4} |
Pentisteriruncitruncated 7-ortoplex (Tecpotaz) | (0,1,2,3,3,4,5)√2 | 645120 | 161280 | ||||||
| 103 |
t 0,2,3,4,5 {3,3,3,3,3,4} |
Pentisteriruncicantellated 7-ortoplex (Tacparez) | (0,1,2,3,4,4,5)√2 | 645120 | 161280 | ||||||
| 104 |
t 1,2,3,4,5 {4,3,3,3,3,3} |
Bisteriruncicanantitruncated 7-cube (Gabcosaz) | (0,1,2,3,4,5,5)√2 | 564480 | 161280 | ||||||
| 105 |
t 0,1,2,3,6 {3,3,3,3,3,4} |
Hexiruncicantitruncated 7-ortoplex (Pugopaz) | (0,0,0,1,2,3,4)√2 + (1,1,1,1,1,1,1) | 483840 | 107520 | ||||||
| 106 |
t 0,1,2,4,6 {3,3,3,3,3,4} |
Hexistericantitruncated 7-ortoplex (Pucagraz) | (0,0,1,1,2,3,4)√2 + (1,1,1,1,1,1,1) | 806400 | 161280 | ||||||
| 107 |
t 0,1,3,4,6 {3,3,3,3,3,4} |
Hexisteriruncitruncated 7-ortoplex (Pucpotaz) | (0,0,1,2,2,3,4)√2 + (1,1,1,1,1,1,1) | 725760 | 161280 | ||||||
| 108 |
t 0,2,3,4,6 {4,3,3,3,3,3} |
Hexisteriruncikantellerad 7-kub (Pucprosaz) | (0,0,1,2,3,3,4)√2 + (1,1,1,1,1,1,1) | 725760 | 161280 | ||||||
| 109 |
t 0,2,3,4,5 {4,3,3,3,3,3} |
Pentisteriruncicantellated 7-kub (Tocpresa) | (0,0,1,2,3,4,4)√2 + (1,1,1,1,1,1,1) | 645120 | 161280 | ||||||
| 110 |
t 0,1,2,5,6 {3,3,3,3,3,4} |
Hexipenticantitruncated 7-ortoplex (Putegraz) | (0,1,1,1,2,3,4)√2 + (1,1,1,1,1,1,1) | 483840 | 107520 | ||||||
| 111 |
t 0,1,3,5,6 {4,3,3,3,3,3} |
Hexipentiruncitruncated 7-kub (Putpetsaz) | (0,1,1,2,2,3,4)√2 + (1,1,1,1,1,1,1) | 806400 | 161280 | ||||||
| 112 |
t 0,1,3,4,6 {4,3,3,3,3,3} |
Hexisteriruncitruncated 7-kub (Pucpetsa) | (0,1,1,2,3,3,4)√2 + (1,1,1,1,1,1,1) | 725760 | 161280 | ||||||
| 113 |
t 0,1,3,4,5 {4,3,3,3,3,3} |
Pentisteriruncitruncated 7-kub (Tecpetsa) | (0,1,1,2,3,4,4)√2 + (1,1,1,1,1,1,1) | 645120 | 161280 | ||||||
| 114 |
t 0,1,2,5,6 {4,3,3,3,3,3} |
Hexipenticantitruncated 7-cube (Putgresa) | (0,1,2,2,2,3,4)√2 + (1,1,1,1,1,1,1) | 483840 | 107520 | ||||||
| 115 |
t 0,1,2,4,6 {4,3,3,3,3,3} |
Hexisterisk antitrunkerad 7-kub (Pucagrosa) | (0,1,2,2,3,3,4)√2 + (1,1,1,1,1,1,1) | 806400 | 161280 | ||||||
| 116 |
t 0,1,2,4,5 {4,3,3,3,3,3} |
Pentistericantitruncated 7-kub (Tecgresa) | (0,1,2,2,3,4,4)√2 + (1,1,1,1,1,1,1) | 645120 | 161280 | ||||||
| 117 |
t 0,1,2,3,6 {4,3,3,3,3,3} |
Hexiruncicantitruncated 7-cube (Pugopsa) | (0,1,2,3,3,3,4)√2 + (1,1,1,1,1,1,1) | 483840 | 107520 | ||||||
| 118 |
t 0,1,2,3,5 {4,3,3,3,3,3} |
Pentiruncicanantitruncated 7-cube (Togapsa) | (0,1,2,3,3,4,4)√2 + (1,1,1,1,1,1,1) | 725760 | 161280 | ||||||
| 119 |
t 0,1,2,3,4 {4,3,3,3,3,3} |
Steriruncicantitruncated 7-cube (Gacosa) | (0,1,2,3,4,4,4)√2 + (1,1,1,1,1,1,1) | 376320 | 107520 | ||||||
| 120 |
t 0,1,2,3,4,5 {3,3,3,3,3,4} |
Pentisteriruncicanantitruncated 7-orthoplex (Gotaz) | (0,1,2,3,4,5,6)√2 | 1128960 | 322560 | ||||||
| 121 |
t 0,1,2,3,4,6 {3,3,3,3,3,4} |
Hexisteriruncicanantitruncated 7-ortoplex (Pugacaz) | (0,0,1,2,3,4,5)√2 + (1,1,1,1,1,1,1) | 1290240 | 322560 | ||||||
| 122 |
t 0,1,2,3,5,6 {3,3,3,3,3,4} |
Hexipentiruncicanantitruncated 7-ortoplex (Putgapaz) | (0,1,1,2,3,4,5)√2 + (1,1,1,1,1,1,1) | 1290240 | 322560 | ||||||
| 123 |
t 0,1,2,4,5,6 {4,3,3,3,3,3} |
Hexipentistericantitrunkerad 7-kub (Putcagrasaz) | (0,1,2,2,3,4,5)√2 + (1,1,1,1,1,1,1) | 1290240 | 322560 | ||||||
| 124 |
t 0,1,2,3,5,6 {4,3,3,3,3,3} |
Hexipentiruncicanantitruncated 7-cube (Putgapsa) | (0,1,2,3,3,4,5)√2 + (1,1,1,1,1,1,1) | 1290240 | 322560 | ||||||
| 125 |
t 0,1,2,3,4,6 {4,3,3,3,3,3} |
Hexisteriruncicanantitruncated 7-cube (Pugacasa) | (0,1,2,3,4,4,5)√2 + (1,1,1,1,1,1,1) | 1290240 | 322560 | ||||||
| 126 |
t 0,1,2,3,4,5 {4,3,3,3,3,3} |
Pentisteriruncicanantitrunkerad 7-kub (Gotesa) | (0,1,2,3,4,5,5)√2 + (1,1,1,1,1,1,1) | 1128960 | 322560 | ||||||
| 127 |
t 0,1,2,3,4,5,6 {4,3,3,3,3,3} |
Omnitruncerad 7-kub (Guposaz) | (0,1,2,3,4,5,6)√2 + (1,1,1,1,1,1,1) | 2257920 | 645120 | ||||||
Familjen D 7
D 7 -familjen har symmetri av ordningen 322560 (7 factorial x 2 6 ).
Denna familj har 3×32−1=95 Wythoffian enhetliga polytoper, genererade genom att markera en eller flera noder i D 7 Coxeter-Dynkin diagrammet . Av dessa är 63 (2×32−1) upprepade från B 7 -familjen och 32 är unika för denna familj, listade nedan. Bowers namn och akronym ges för korsreferenser.
Se även lista över D7-polytoper för Coxeter-plangrafer för dessa polytoper.
| D 7 enhetliga polytoper | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| # | Coxeter diagram | Namn |
Baspunkt (alternativt signerad) |
Element räknas | |||||||
| 6 | 5 | 4 | 3 | 2 | 1 | 0 | |||||
| 1 |
|
7-kuber demihepteract (hesa) |
(1,1,1,1,1,1,1) | 78 | 532 | 1624 | 2800 | 2240 | 672 | 64 | |
| 2 |
|
cantic 7-kub trunkerad demihepteract (thesa) |
(1,1,3,3,3,3,3) | 142 | 1428 | 5656 | 11760 | 13440 | 7392 | 1344 | |
| 3 |
|
runkad 7-kub liten romberad demiheptera (sirhesa) |
(1,1,1,3,3,3,3) | 16800 | 2240 | ||||||
| 4 |
|
sterisk 7-kub liten prismatad demihepteract (sphosa) |
(1,1,1,1,3,3,3) | 20160 | 2240 | ||||||
| 5 |
|
pentic 7-kuber småcellig demihepteract (sochesa) |
(1,1,1,1,1,3,3) | 13440 | 1344 | ||||||
| 6 |
|
hexisk 7-kub liten terated demihepteract (suthesa) |
(1,1,1,1,1,1,3) | 4704 | 448 | ||||||
| 7 |
|
runcicantic 7-kub stor romberad demihepteract (Girhesa) |
(1,1,3,5,5,5,5) | 23520 | 6720 | ||||||
| 8 |
|
sterikantisk 7-kubbar prismatotrunkerad demihepteract (pothesa) |
(1,1,3,3,5,5,5) | 73920 | 13440 | ||||||
| 9 |
|
steriruncisk 7-kubbar prismatorhomatiserad demihepterakt (prohesa) |
(1,1,1,3,5,5,5) | 40320 | 8960 | ||||||
| 10 |
|
penticantisk 7-kubbar cellitruncated demihepteract (cothesa) |
(1,1,3,3,3,5,5) | 87360 | 13440 | ||||||
| 11 |
|
pentiruncic 7-kubbar cellirhomberad demihepteract (crohesa) |
(1,1,1,3,3,5,5) | 87360 | 13440 | ||||||
| 12 |
|
pentisterisk 7-kubbar celliprismatad demihepteract (caphesa) |
(1,1,1,1,3,5,5) | 40320 | 6720 | ||||||
| 13 |
|
hexicantic 7-cube tericantic demihepteract (tuthesa) |
(1,1,3,3,3,3,5) | 43680 | 6720 | ||||||
| 14 |
|
hexiruncic 7-kuber terirhombated demihepteract (turhesa) |
(1,1,1,3,3,3,5) | 67200 | 8960 | ||||||
| 15 |
|
hexisterisk 7-kuber teriprismatad demiheptera (tuphesa) |
(1,1,1,1,3,3,5) | 53760 | 6720 | ||||||
| 16 |
|
hexipentisk 7-kub- tericellerad demihepteract (tuchesa) |
(1,1,1,1,1,3,5) | 21504 | 2688 | ||||||
| 17 |
|
steriruncicantic 7-kub stor prismatad demihepteract (Gephosa) |
(1,1,3,5,7,7,7) | 94080 | 26880 | ||||||
| 18 |
|
pentiruncicantic 7-kubbar celligreatorhombated demihepteract (cagrohesa) |
(1,1,3,5,5,7,7) | 181440 | 40320 | ||||||
| 19 |
|
pentisterikantisk 7-kubbar celliprismatotruncerad demihepteract (capthesa) |
(1,1,3,3,5,7,7) | 181440 | 40320 | ||||||
| 20 |
|
pentisteriruncic 7-kubbar celliprismatorhomberad demihepteract (coprahesa) |
(1,1,1,3,5,7,7) | 120960 | 26880 | ||||||
| 21 |
|
hexiruncicantic 7-kuber terigreatorhombated demihepteract (tugrohesa) |
(1,1,3,5,5,5,7) | 120960 | 26880 | ||||||
| 22 |
|
hexisterikantisk 7-kub teriprismat till trunkad demihepterakt (tupthesa) |
(1,1,3,3,5,5,7) | 221760 | 40320 | ||||||
| 23 |
|
hexisteriruncic 7-kuber teriprismatorhombated demihepteract (tuprohesa) |
(1,1,1,3,5,5,7) | 134400 | 26880 | ||||||
| 24 |
|
hexipenticantic 7-kube teriCellitruncated demiheptera (tucothesa) |
(1,1,3,3,3,5,7) | 147840 | 26880 | ||||||
| 25 |
|
hexipentiruncic 7-kuber tericellirhombated demihepteract (tucrohesa) |
(1,1,1,3,3,5,7) | 161280 | 26880 | ||||||
| 26 |
|
hexipentisterisk 7-kub tericelliprismatad demiheptera (tucophesa) |
(1,1,1,1,3,5,7) | 80640 | 13440 | ||||||
| 27 |
|
pentisteriruncicantic 7-kub stor cellad demihepteract (gochesa) |
(1,1,3,5,7,9,9) | 282240 | 80640 | ||||||
| 28 |
|
hexisteriruncicantic 7-kuber terigreatoprimerad demihepteract (tugphesa) |
(1,1,3,5,7,7,9) | 322560 | 80640 | ||||||
| 29 |
|
hexipentiruncicantic 7-kuber tericelligreatorhombated demihepteract (tucagrohesa) |
(1,1,3,5,5,7,9) | 322560 | 80640 | ||||||
| 30 |
|
hexipentistericantic 7-kuber tericelliprismatotruncated demihepteract (tucpathesa) |
(1,1,3,3,5,7,9) | 362880 | 80640 | ||||||
| 31 |
|
hexipentisteriruncic 7-kuber tericellprismatorhombated demihepteract (tucprohesa) |
(1,1,1,3,5,7,9) | 241920 | 53760 | ||||||
| 32 |
|
hexipentisteriruncicantic 7-kub stor terated demihepteract (guthesa) |
(1,1,3,5,7,9,11) | 564480 | 161280 | ||||||
Familjen E 7
E 7 Coxeter-gruppen har order 2 903 040.
Det finns 127 former baserade på alla permutationer av Coxeter-Dynkin-diagrammen med en eller flera ringar.
Se även en lista över E7-polytoper för symmetriska Coxeter-plangrafer för dessa polytoper.
| E 7 enhetliga polytoper | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| # |
Coxeter-Dynkin diagram Schläfli symbol |
Namn | Element räknas | ||||||||
| 6 | 5 | 4 | 3 | 2 | 1 | 0 | |||||
| 1 | 2 31 (laq) | 632 | 4788 | 16128 | 20160 | 10080 | 2016 | 126 | |||
| 2 | Rättad 2 31 (rolaq) | 758 | 10332 | 47880 | 100800 | 90720 | 30240 | 2016 | |||
| 3 | Rättad 1 32 (rolin) | 758 | 12348 | 72072 | 191520 | 241920 | 120960 | 10080 | |||
| 4 | 1 32 (lin) | 182 | 4284 | 23688 | 50400 | 40320 | 10080 | 576 | |||
| 5 | Birectified 3 21 (branq) | 758 | 12348 | 68040 | 161280 | 161280 | 60480 | 4032 | |||
| 6 | Rättad 3 21 (ranq) | 758 | 44352 | 70560 | 48384 | 11592 | 12096 | 756 | |||
| 7 | 3 21 (naq) | 702 | 6048 | 12096 | 10080 | 4032 | 756 | 56 | |||
| 8 | Trunkerad 231 (talq) | 758 | 10332 | 47880 | 100800 | 90720 | 32256 | 4032 | |||
| 9 | Kantellerad 231 (sirlaq) | 131040 | 20160 | ||||||||
| 10 | Bitruncated 2 31 (botlaq) | 30240 | |||||||||
| 11 | liten avskalad 2 31 (shilq) | 2774 | 22428 | 78120 | 151200 | 131040 | 42336 | 4032 | |||
| 12 | avriktad 2 31 (hirlaq) | 12096 | |||||||||
| 13 | trunkerad 1 32 (tolin) | 20160 | |||||||||
| 14 | liten demiprismatad 2 31 (shiplaq) | 20160 | |||||||||
| 15 | birectified 1 32 (berlin) | 758 | 22428 | 142632 | 403200 | 544320 | 302400 | 40320 | |||
| 16 | tritruncerad 3 21 (totanq) | 40320 | |||||||||
| 17 | demibirectified 3 21 (hobranq) | 20160 | |||||||||
| 18 | liten cell 2 31 (scalq) | 7560 | |||||||||
| 19 | liten biprismat 2 31 (sobpalq) | 30240 | |||||||||
| 20 | liten birhombated 3 21 (sabranq) | 60480 | |||||||||
| 21 | avriktad 3 21 (harnaq) | 12096 | |||||||||
| 22 | bitruncated 3 21 (botnaq) | 12096 | |||||||||
| 23 | liten terated 3 21 (stanq) | 1512 | |||||||||
| 24 | liten demicellad 3 21 (shocanq) | 12096 | |||||||||
| 25 | liten prismatad 3 21 (spanq) | 40320 | |||||||||
| 26 | liten avskalad 3 21 (shanq) | 4032 | |||||||||
| 27 | liten rhomberad 3 21 (sranq) | 12096 | |||||||||
| 28 | Trunkerad 321 (tanq) | 758 | 11592 | 48384 | 70560 | 44352 | 12852 | 1512 | |||
| 29 | great rhombated 2 31 (girlaq) | 60480 | |||||||||
| 30 | demitruncated 2 31 (hotlaq) | 24192 | |||||||||
| 31 | liten demirhomberad 2 31 (sherlaq) | 60480 | |||||||||
| 32 | demibitruncated 2 31 (hobtalq) | 60480 | |||||||||
| 33 | demiprismatad 2 31 (hiptalq) | 80640 | |||||||||
| 34 | demiprismatorhombated 2 31 (hiprolaq) | 120960 | |||||||||
| 35 | bitruncated 1 32 (batlin) | 120960 | |||||||||
| 36 | liten prismatad 2 31 (spalq) | 80640 | |||||||||
| 37 | liten rhomberad 1 32 (sirlin) | 120960 | |||||||||
| 38 | tritruncerad 2 31 (tatilq) | 80640 | |||||||||
| 39 | cellitruncated 2 31 (catalaq) | 60480 | |||||||||
| 40 | cellirhombated 2 31 (crilq) | 362880 | |||||||||
| 41 | biprismatotruncated 2 31 (biptalq) | 181440 | |||||||||
| 42 | liten prismatad 1 32 (seplin) | 60480 | |||||||||
| 43 | liten biprismatad 3 21 (sabipnaq) | 120960 | |||||||||
| 44 | liten demibirhombated 3 21 (shobranq) | 120960 | |||||||||
| 45 | cellidemiprismated 2 31 (chaplaq) | 60480 | |||||||||
| 46 | demibiprismatotruncated 3 21 (hobpotanq) | 120960 | |||||||||
| 47 | great birhombated 3 21 (gobranq) | 120960 | |||||||||
| 48 | demibitruncated 3 21 (hobtanq) | 60480 | |||||||||
| 49 | teritruncated 2 31 (totalq) | 24192 | |||||||||
| 50 | terirhombated 2 31 (trilq) | 120960 | |||||||||
| 51 | demicelliprismated 3 21 (hicpanq) | 120960 | |||||||||
| 52 | liten teridemifierad 2 31 (sethalq) | 24192 | |||||||||
| 53 | liten cell 3 21 (scanq) | 60480 | |||||||||
| 54 | demiprismated 3 21 (hipnaq) | 80640 | |||||||||
| 55 | terirhombated 3 21 (tranq) | 60480 | |||||||||
| 56 | demicellirhombated 3 21 (hocranq) | 120960 | |||||||||
| 57 | prismatorhombated 3 21 (pranq) | 120960 | |||||||||
| 58 | liten demirhomberad 3 21 (sharnaq) | 60480 | |||||||||
| 59 | teritruncated 3 21 (tetanq) | 15120 | |||||||||
| 60 | demicellitruncated 3 21 (hictanq) | 60480 | |||||||||
| 61 | prismatotruncated 3 21 (potanq) | 120960 | |||||||||
| 62 | demitruncated 3 21 (hotnaq) | 24192 | |||||||||
| 63 | stor rhombated 3 21 (granq) | 24192 | |||||||||
| 64 | stor förnedrad 2 31 (gahlaq) | 120960 | |||||||||
| 65 | great demiprismated 2 31 (gahplaq) | 241920 | |||||||||
| 66 | prismatotruncated 2 31 (potlaq) | 241920 | |||||||||
| 67 | prismatorhombated 2 31 (prolaq) | 241920 | |||||||||
| 68 | great rhombated 1 32 (girlin) | 241920 | |||||||||
| 69 | celligreatorhombated 2 31 (cagrilq) | 362880 | |||||||||
| 70 | cellidemitruncated 2 31 (chotalq) | 241920 | |||||||||
| 71 | prismatotruncated 1 32 (patlin) | 362880 | |||||||||
| 72 | biprismatorhombated 3 21 (bipirnaq) | 362880 | |||||||||
| 73 | tritruncerad 1 32 (tatlin) | 241920 | |||||||||
| 74 | cellidemiprismatorhombated 2 31 (chopralq) | 362880 | |||||||||
| 75 | great demibiprismated 3 21 (ghobipnaq) | 362880 | |||||||||
| 76 | celliprismatad 2 31 (caplaq) | 241920 | |||||||||
| 77 | biprismatotruncated 3 21 (boptanq) | 362880 | |||||||||
| 78 | great trirhombated 2 31 (gatralaq) | 241920 | |||||||||
| 79 | terigreatorhombated 2 31 (togrilq) | 241920 | |||||||||
| 80 | teridemitruncated 2 31 (thotalq) | 120960 | |||||||||
| 81 | teridemirhombated 2 31 (thorlaq) | 241920 | |||||||||
| 82 | celliprismated 3 21 (capnaq) | 241920 | |||||||||
| 83 | teridemiprismatotruncated 2 31 (thoptalq) | 241920 | |||||||||
| 84 | teriprismatorhombated 3 21 (tapronaq) | 362880 | |||||||||
| 85 | demicelliprismatorhombated 3 21 (hacpranq) | 362880 | |||||||||
| 86 | teriprismated 2 31 (toplaq) | 241920 | |||||||||
| 87 | cellirhombated 3 21 (cranq) | 362880 | |||||||||
| 88 | demiprismatorhombated 3 21 (hapranq) | 241920 | |||||||||
| 89 | tericellitruncated 2 31 (tectalq) | 120960 | |||||||||
| 90 | teriprismatotruncated 3 21 (toptanq) | 362880 | |||||||||
| 91 | demicelliprismatotruncated 3 21 (hecpotanq) | 362880 | |||||||||
| 92 | teridemitruncated 3 21 (thotanq) | 120960 | |||||||||
| 93 | cellitruncated 3 21 (catnaq) | 241920 | |||||||||
| 94 | demiprismatotruncated 3 21 (hiptanq) | 241920 | |||||||||
| 95 | terigreatorhombated 3 21 (tagranq) | 120960 | |||||||||
| 96 | demicelligreatorhombated 3 21 (hicgarnq) | 241920 | |||||||||
| 97 | great prismated 3 21 (gopanq) | 241920 | |||||||||
| 98 | stora demirhomberade 3 21 (gahranq) | 120960 | |||||||||
| 99 | great prismated 2 31 (gopalq) | 483840 | |||||||||
| 100 | stor cellidimifierad 2 31 (gechalq) | 725760 | |||||||||
| 101 | great birhombated 1 32 (gebrolin) | 725760 | |||||||||
| 102 | prismatorhombated 1 32 (prolin) | 725760 | |||||||||
| 103 | celliprismatorhombated 2 31 (caprolaq) | 725760 | |||||||||
| 104 | great biprismated 2 31 (gobpalq) | 725760 | |||||||||
| 105 | tericelliprismated 3 21 (ticpanq) | 483840 | |||||||||
| 106 | teridemigreatoprismated 2 31 (thegpalq) | 725760 | |||||||||
| 107 | teriprismatotruncated 2 31 (teptalq) | 725760 | |||||||||
| 108 | teriprismatorhombated 2 31 (topralq) | 725760 | |||||||||
| 109 | cellipriemsatorhombated 3 21 (copranq) | 725760 | |||||||||
| 110 | tericelligreatorhombated 2 31 (tecgrolaq) | 725760 | |||||||||
| 111 | tericellitruncated 3 21 (tectanq) | 483840 | |||||||||
| 112 | teridemiprismatotruncated 3 21 (thoptanq) | 725760 | |||||||||
| 113 | celliprismatotruncated 3 21 (coptanq) | 725760 | |||||||||
| 114 | teridemicelligreatorhombated 3 21 (thocgranq) | 483840 | |||||||||
| 115 | terigreatoprismated 3 21 (tagpanq) | 725760 | |||||||||
| 116 | great demicellated 3 21 (gahcnaq) | 725760 | |||||||||
| 117 | tericelliprismaterad laq (tecpalq) | 483840 | |||||||||
| 118 | celligreatorhombated 3 21 (cogranq) | 725760 | |||||||||
| 119 | stor förnedrad 3 21 (gahnq) | 483840 | |||||||||
| 120 | great cellated 2 31 (gocalq) | 1451520 | |||||||||
| 121 | terigreatoprismated 2 31 (tegpalq) | 1451520 | |||||||||
| 122 | tericelliprismatotruncated 3 21 (tecpotniq) | 1451520 | |||||||||
| 123 | tericellidemigreatoprismated 2 31 (techogaplaq) | 1451520 | |||||||||
| 124 | tericelligreatorhombated 3 21 (tacgarnq) | 1451520 | |||||||||
| 125 | tericelliprismatorhombated 2 31 (tecprolaq) | 1451520 | |||||||||
| 126 | great cellated 3 21 (gocanq) | 1451520 | |||||||||
| 127 | stor terated 3 21 (gotanq) | 2903040 | |||||||||
Regelbundna och enhetliga bikakor
Det finns fem grundläggande affina Coxeter-grupper och sexton prismatiska grupper som genererar regelbundna och enhetliga tesselleringar i 6-rum:
| # | Coxeter grupp | Coxeter diagram | Blanketter | |
|---|---|---|---|---|
| 1 | [3 [7] ] | 17 | ||
| 2 | [4,3 4 ,4] | 71 | ||
| 3 |
h[4,3 4 ,4] [4,3 3 ,3 1,1 ] |
95 (32 nya) | ||
| 4 |
q[4,3 4 ,4] [3 1,1 ,3 2 ,3 1,1 ] |
41 (6 nya) | ||
| 5 | [3 2,2,2 ] | 39 | ||
Regelbundna och enhetliga tesseller inkluderar:
-
, 17 former
- Uniform 6-simplex honeycomb : {3 [7] }









- Uniform cyklotrunkerad 6-simplex honungskaka : t 0,1 {3 [7] }








- Uniform Omnitruncated 6-simplex honeycomb : t 0,1,2,3,4,5,6,7 {3 [7] }








- Uniform 6-simplex honeycomb : {3 [7] }
-
, [4,3 4 ,4], 71 former
- Regelbunden 6-kubbar honeycomb , representerad av symbolerna {4,3 4 ,4},













- Regelbunden 6-kubbar honeycomb , representerad av symbolerna {4,3 4 ,4},
-
, [3 1,1 ,3 3 ,4], 95 former, 64 delade med , 32 nya
-












 Uniform 6-demicube honeycomb , representerade av symbolerna h{4,3 4 ,4} = {3 1,1 ,3 3 ,4}, =
Uniform 6-demicube honeycomb , representerade av symbolerna h{4,3 4 ,4} = {3 1,1 ,3 3 ,4}, = 










-
-
, [3 1,1 ,3 2 ,3 1,1 ], 41 unika ringade permutationer, mest delade med och och 6 är nya. Coxeter kallar den första för en kvarts 6-kubisk honeycomb .
-








 =
=












-








 =
=












-








 =
=












-








 =
=












-








 =
=












-








 =
=












-
-
: [3 2,2,2 ], 39 former
- Uniform 2 22 honeycomb : representeras av symbolerna {3,3,3 2,2 },









- Uniform t 4 (2 22 ) honeycomb: 4r{3,3,3 2,2 },









- Uniform 0 222 honeycomb: {3 2,2,2 },









- Uniform t 2 (0 222 ) honeycomb: 2r{3 2,2,2 },









- Uniform 2 22 honeycomb : representeras av symbolerna {3,3,3 2,2 },
| # | Coxeter grupp | Coxeter-Dynkin diagram | |
|---|---|---|---|
| 1 | x | [3 [6] , 2,∞] |
|
| 2 | x | [4,3,3 1,1 ,2,∞] |
|
| 3 | x | [4,3 3 ,4,2,∞] |
|
| 4 | x | [3 1,1 ,3,3 1,1 ,2,∞] |
|
| 5 | x x | [3 [5] ,2,∞,2,∞,2,∞] |
|
| 6 | x x | [4,3,3 1,1 ,2,∞,2,∞] |
|
| 7 | x x | [4,3,3,4,2,∞,2,∞] |
|
| 8 | x x | [3 1,1,1,1 ,2,∞,2,∞] |
|
| 9 | x x | [3,4,3,3,2,∞,2,∞] |
|
| 10 | x x x | [4,3,4,2,∞,2,∞,2,∞] |
|
| 11 | x x x | [4,3 1,1 ,2,∞,2,∞,2,∞] |
|
| 12 | x x x | [3 [4] ,2,∞,2,∞,2,∞] |
|
| 13 | x x x x | [4,4,2,∞,2,∞,2,∞,2,∞] |
|
| 14 | x x x x | [6,3,2,∞,2,∞,2,∞,2,∞] |
|
| 15 | x x x x | [3 [3] ,2,∞,2,∞,2,∞,2,∞] |
|
| 16 | x x x x x | [∞,2,∞,2,∞,2,∞,2,∞] |
|
Regelbundna och enhetliga hyperboliska bikakor
Det finns inga kompakta hyperboliska Coxeter-grupper av rang 7, grupper som kan generera bikakor med alla ändliga fasetter och en finit vertexfigur . Det finns dock 3 parakompakta hyperboliska Coxeter-grupper av rang 7, som var och en genererar enhetliga bikakor i 6-rum som permutationer av ringar i Coxeter-diagrammen.
|
= [3,3 [6] ]: |
= [3 1,1 ,3,3 2,1 ]: |
= [4,3,3,3 2,1 ]: |
Anteckningar om Wythoff-konstruktionen för de enhetliga 7-polytoperna
De reflekterande 7-dimensionella likformiga polytoperna är konstruerade genom en Wythoff-konstruktionsprocess och representeras av ett Coxeter-Dynkin-diagram, där varje nod representerar en spegel. En aktiv spegel representeras av en ringad nod. Varje kombination av aktiva speglar genererar en unik enhetlig polytop. Uniforma polytoper benämns i förhållande till de vanliga polytoperna i varje familj. Vissa familjer har två vanliga konstruktörer och kan därför namnges på två lika giltiga sätt.
Här är de primära operatorerna som är tillgängliga för att konstruera och namnge de enhetliga 7-polytoperna.
De prismatiska formerna och förgrenade graferna kan använda samma trunkeringsindexeringsnotation, men kräver ett explicit numreringssystem på noderna för tydlighetens skull.
| Drift |
Utökad Schläfli-symbol |
Coxeter- Dynkin diagram |
Beskrivning |
|---|---|---|---|
| Förälder | 0 t {p,q,r,s,t,u} |
|
Vilken vanlig 7-polytop som helst |
| Rättad till | t 1 {p,q,r,s,t,u} |
|
Kanterna är helt trunkerade till enstaka punkter. 7-polytopen har nu de kombinerade ansiktena av förälder och dubbel. |
| Birectified | t 2 {p,q,r,s,t,u} |
|
Birektifiering reducerar celler till sina dualer . |
| Trunkerad | t 0,1 {p,q,r,s,t,u} |
|
Varje ursprunglig vertex skärs av, med ett nytt ansikte som fyller gapet. Trunkering har en grad av frihet, som har en lösning som skapar en enhetlig trunkerad 7-polytop. 7-polytopen har sina ursprungliga ytor dubblerade på sidorna och innehåller sidorna av dualen.
|
| Bitruncated | t 1,2 {p,q,r,s,t,u} |
|
Bitrunction omvandlar celler till sin dubbla trunkering. |
| Tritrunkerad | t 2,3 {p,q,r,s,t,u} |
|
Tritrunkering omvandlar 4-faces till deras dubbla trunkering. |
| Kantellerad | t 0,2 {p,q,r,s,t,u} |
|
Förutom vertexstympning är varje originalkant avfasad med nya rektangulära ytor som dyker upp på deras plats. En enhetlig kantellation är halvvägs mellan både den överordnade och dubbla formen. 
|
| Tvåkantigt | t 1,3 {p,q,r,s,t,u} |
|
Förutom vertexstympning är varje originalkant avfasad med nya rektangulära ytor som dyker upp på deras plats. En enhetlig kantellation är halvvägs mellan både den överordnade och dubbla formen. |
| Runcinerad | t 0,3 {p,q,r,s,t,u} |
|
Runcination reducerar celler och skapar nya celler vid hörn och kanter. |
| Biruncinerad | t 1,4 {p,q,r,s,t,u} |
|
Runcination reducerar celler och skapar nya celler vid hörn och kanter. |
| Sterikerad | t 0,4 {p,q,r,s,t,u} |
|
Sterikering minskar 4-ytor och skapar nya 4-ytor vid hörn, kanter och ytor i mellanrummen. |
| Pentellerad | t 0,5 {p,q,r,s,t,u} |
|
Pentellation minskar 5-ytor och skapar nya 5-ytor vid hörn, kanter, ytor och celler i mellanrummen. |
| Hexicerad | t 0,6 {p,q,r,s,t,u} |
|
Hexikation minskar 6-ytor och skapar nya 6-ytor vid hörn, kanter, ytor, celler och 4-ytor i mellanrummen. ( expansionsoperation för 7-polytoper) |
| Omnitruncerad | t 0,1,2,3,4,5,6 {p,q,r,s,t,u} |
|
Alla sex operatorerna trunkering, kantellation, runcination, sterikering, pentellation och hexication tillämpas. |
- T. Gosset : On the Regular and Semi-Regular Figures in Space of n Dimensions , Messenger of Mathematics , Macmillan, 1900
- A. Boole Stott : Geometrisk deduktion av halvregelbundna från vanliga polytoper och rymdfyllningar , Verhandelingen av Koninklijke academy van Wetenschappen width unit Amsterdam, Eerste Sectie 11,1, Amsterdam, 1910
-
HSM Coxeter :
- HSM Coxeter, MS Longuet-Higgins och JCP Miller: Uniform Polyhedra , Philosophical Transactions of the Royal Society of London, London, 1954
- HSM Coxeter, Regular Polytopes , 3:e upplagan, Dover New York, 1973
-
Kaleidoscopes: Selected Writings of HSM Coxeter , redigerad av F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6 http://www. wiley.com/WileyCDA/WileyTitle/productCd-0471010030.html
- (Papper 22) HSM Coxeter, Regular and Semi Regular Polytopes I , [Math. Zeit. 46 (1940) 380-407, MR 2,10]
- (Papper 23) HSM Coxeter, Regular and Semi-Regular Polytopes II , [Math. Zeit. 188 (1985) 559-591]
- (Papper 24) HSM Coxeter, Regular and Semi-Regular Polytopes III , [Math. Zeit. 200 (1988) 3-45]
- NW Johnson : Theory of Uniform Polytopes and Honeycombs , Ph.D. Avhandling, University of Toronto, 1966
- Klitzing, Richard. "7D enhetliga polytoper (polyexa)" .